Celtic Garland
Number Theory
Level
2
How many distinct ropes is this garland made out of?
(Hint: You can carefully trace though the entire picture, OR, there is a very fast mathematical trick for learning the answer.)
8
1
2
4
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
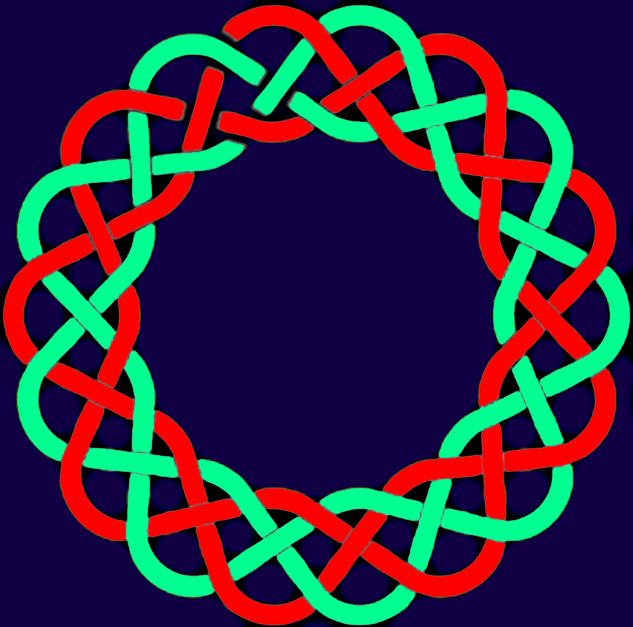
We see that at each 'point' in the 'circle', there are 4 'pieces' of rope intersecting. One at the peak, one at the trough, one going up, one going down. Counting the total number of peaks in the picture, there are 18. The least common multiple of those two numbers is 36, twice of 18, which tells you each rope loops twice before ending up at the starting point. Since each 'point' has 4 intersecting ropes, and each rope loops twice, you can infer that there are two ropes, each looping twice, thereby forming the 4 intersecting ropes at each point.