Chain Reaction - 2
One day Alice and Bob were terribly bored, so they decided to play a game of
Chain Reaction
. Once they started playing, they were having so much fun that they didn't want the game to end.
What is the maximum number of moves they could have made in a single game?
Also try Part 1
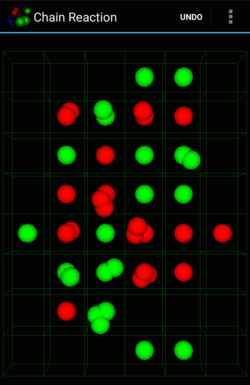
- A move is defined as placing an orb in the grid. As an explicit example, if Alice places an orb, and then Bob places an orb, then they have played two moves. The final orb which is placed, due to which one player wins is also to be counted.
- You may want to read the rules of Chain Reaction first. To understand the rules better, it is recommended that you try playing the game by downloading it from the Play Store .
- Alice and Bob were both playing with the objective to maximize the number of moves in the game.
- They were playing in a grid, similar to the one in the above image.
- Alice and Bob are very smart.
The answer is 117.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
According to the logic of the game, the general formula for (m * n) grid is:
(m-2) * (n-2) * (3) + (m+n-3) * (4) + 1 = Tn = maximum number of moves:
The above formula can be explained as follows: As the corner can have 1 orb before split, sides can have 2, and the rest of others have 3 before split..and 1 last move for split off..other cases does not guarantee the existence of both till end and maximum number of moves.
Enjoy playing the game!