Change Is The Only Constant Thing On The Sea - Part 1

Calculus Level 3

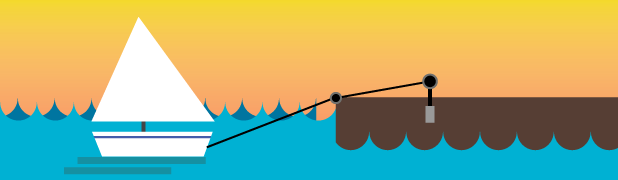When ships come in to dock where the berths are extremely narrow, the ships need to be towed in to prevent damage to both the dock and the ship. A metal cable is attached to the front of the ship, looped through a pulley that is hinged at the edge of the dock, and attached to an automated winch.

If the automated winch winds the cable at a constant rate, which of the following describes the speed at which the boat will be pulled in (prior to reaching the dock)?

Speed increases over time Speed decreases over time Speed is constant Speed varies too much, no simple description

View wiki
Chung Gene Keun by Chung Gene Keun , 42, USA

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

1 solution

Discussions for this problem are now closed

Maharnab Mitra
May 11, 2014

mm mm

Let speed of pulling the cable be v v .

y 2 = x 2 + c 2 ⟹ 2 y d y d t = 2 x d x d t ⟹ y x v = d x d t ⟹ v s i n θ = d x d t y^2=x^2+c^2 \implies 2y \frac{dy}{dt} =2x \frac{dx}{dt} \implies \frac{y}{x} v =\frac{dx}{dt} \implies \frac{v}{sin \theta} = \frac{dx}{dt}

As θ \theta decreases, s i n θ sin \theta decreases and as a result v s i n θ \frac{v}{sin \theta} increases. Thus, speed of boat, i.e., d x d t \frac{dx}{dt} increases over time.

19 Helpful 0 Interesting 0 Brilliant 0 Confused

Most people think that the boat can only move less than the change in the distance moved by the rope. However, this is not true, and we can demonstrate it as such:

Let D D be the point on the dock. Let X 1 X_1 be the initial point on the boat, let X 2 X_2 be the final point on the boat after it moved for 1 second. The problem tells us that D X 1 − D X 2 DX_1 - DX_2 is the contraction of the length of the rope.

Hence, by the triangle inequality, we get that X 1 X 2 + D X 2 > D X 1 X_1 X_2 + DX_2 > DX_1 or that X 1 X 2 > D X 1 − D X 2 = 10 X_1 X_2 > DX_1 - DX_2 = 10 . Hence, the boat moves more the contraction of the length of the rope.

Chung Gene Keun - 7 years, 1 month ago

How do you know D X 1 − D X 2 = 10 DX_1-DX_2 =10 ?

Maharnab Mitra - 7 years, 1 month ago

Sorry, I used that value in the other question. What I should have said was X 1 X 2 X_1 X_2 is the distance that the boat moved, which is greater than the speed at which the cable is pulled in. Edited my previous comment.

Chung Gene Keun - 7 years ago

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...