Checkmate in 1
In the following position, how can checkmate be given in 1 move?
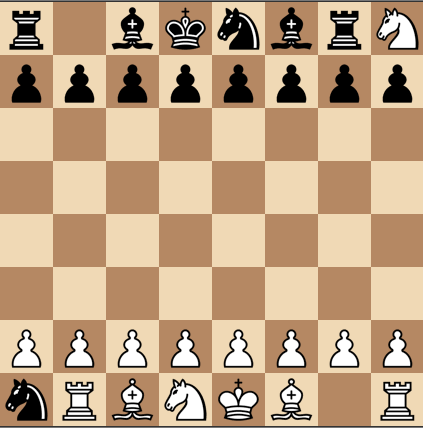
If the bottom left corner is denoted by ( 1 , 1 ) , what is the target square of the last move?
- This game starts from the standard position, and we arrived at this position using the standard rules of chess.
- You have to determine who is to play.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
Great detailed analysis of the problem!
The problem here is that, even though your analysis assumes minimal number of moves to reach the position, it does not say so. Regular chess puzzles usually assume it's white to move, unless stated otherwise (such as a historical game).
If someone from brilliant.org sees this, please make this adjustment to your chess puzzles. It's the standard. It's rather rude to not state who is to move, especially when either could give checkmate in one.
Log in to reply
Thanks for the feedback. We are currently evaluating how best to present chess puzzles, given the myriad of (sometimes random) rules that could apply. I agree that providing more guidance about the setup would help others appreciate approaching the problem.
I've just edited the problem. What do you think of the current phrasing?
It is the standard in the chess world, but especially in the puzzling community (and not the chess community) there are a lot of trick positions, where you need to figure out what has occurred previously in order to find the solution. As an example, a book full of such problems is The Chess Mysteries of Sherlock Holmes by Raymond Smullyan.
If you will notice, when a chess game starts, thr black queen is on the black square, fhe white queen on the ahite square. As such, either the setup is wrong, meaning neither has moved, or they have both moved, thus there is an equality in the number of moves. As such, it is whites turn to move.
Log in to reply
That argument is based on the assumption that the initial starting position is rotationally symmetric. However, that assumption is not true. It is only reflection symmetric, and in particular, the kings (and queens) start off in the same column.
Nice. I would just add: anytime that White's [resp. Black's] pawns and bishops are all in their respective original positions, it follows that every move White has made was either from a white square to a black square or vice versa.
If it is also the case that White's rooks and knights are all uncaptured, then one can determine whether White has had an odd or even number of turns based on the current position. (The parity of the number of turns White has had is equal to the parity of the number of white pieces on black squares.)
Similarly for Black.
(In this case, there are 7 white pieces on black squares, and 8 black pieces on white squares, meaning that White and Black cannot have had the same number of moves.)
Log in to reply
Just for the heck of it, let me post a question of my own here.
I laid out above that we can determine whether White has had an odd or even number of turns based on the current position, provided that White has not moved any pawn or lost any piece except possibly the queen. But can we determine whether White has had an odd or even number of turns based on the current position, on any board that does satisfy those 2 conditions?
The two possible moves are obvious, where the product is either 2 or 30, depending on who has to move first.
First let's consider the pawns and the bisshops. the pawns didn't move at all, as well as the bisshops, so they can't count as moves.
Next the knights. We know that all knights hop from a light square to a dark square and vice versa. By the symmetry of the board you can tell that black has made as many moves as white.
Now the rooks and the queens. Rooks and queens can normally make uneven moves and come back to its original position, but not in this case. They are (or were) both restricted (by the pawns and the bisshops) so that they cannot do things like that. Thereby, and also by the symmetry of the board, we can make the same conclusion as with the knights.
Considering neither white nor black made a king move, it should be white to move. But we can see that the white king is not on its original position, and the black king is. Both kings are restricted, enough to prove that white has made a move mores than black, so the final product is 2.
Actually, There are no queens so capturing must be considered and it is the black king that has moved, not the white king. The bottom right corner is white as it should be which means white king starts on a black square and the black king starts on a white square. therefore, it should be white's move
Why the answer is not (5,6) = 30?
Yes, why not? Black and white knights have been moved an even number, so it is white's turn. If it were black's turn, black would have been moved an odd number and white an even, or vice versa. It is white's turn. Answer should be 30.
I tried that too. Maybe there is no combination of moves that lead to this position while giving white the last move.
Log in to reply
Is it mathematically possible for the board to be reverse-mirror-imaged and for white to have made one more move than black?
Ah, I've got it.
On the face of it, black has made one more move than white, but that's not possible.
Therefore, White has moved his King twice before moving his other Knight to d1, and it's black to move, and 1 ... Nc2 is correct.
Maybe it was supposed to be the top right corner?
I got my answer by moving the black knight in the bottom left over two and up one. This is a one move victory for black. He counts the squares with the first one being 0 so the destination is (2,1)
I consider this chessboard set an impossible one. Of course, by the movements we can have this set, but only if it was agreed between the players to do so. To capture the Queen (the only piece missing in both sides), the Knight would have to be exposed to at least one of the Pawns, and at least twice (going into and out of the Pawn line). And I'm not even counting the useless Rook move.
Hey, I wrote a solution describing exactly why this cannot be the case, with a sample game to prove it.
Checkmate cannot be achieved in one move, if you move a knight to place the king in check the knight can then be taken by a pawn therefore there is no checkmate
Note: saying the "bottom, left corner is (1,1)" doesn't remove the ambiguity of whether that means (bottom,left) or (left,bottom). In a standard x-y notation it would be (left,bottom) but in matrix row-column notation in would be (bottom,left). That the text puts bottom first is a bit misleading.
They have told to start from bottom left corner, so it must be black's turn, though I dont know how.
It may seem like white to move. This is due to the assumption that white and black have moved the same number of times, in which case it would indeed be white to move. However, note that the black king has moved one square.
Here is a sample game that would result in this position.
Thus the position, with Black to move.
It has been established that it is possible to reach the above position with black to move. If there exists a method of switching this by making 2 moves, accomplishing something that could have been done in 1, then it could be white to move.
Since there exists no method to "give a free move" to the opponent here, it must be black to move. Therefore, the mate in one in question must be: ..Nxc2#. The coordinates of c2 by the given system is (1, 2), and 1 * 2 = 2.
A cleaner solution is to simply look at the even and odds.
For white, we must consider whether it takes an odd or even number of moves for the kingside Knight to reach h8.
A hashtag (#) represents a Black piece that has made an odd number of moves. There are 4. A percent (%) represents a White piece that has made an odd number of moves. There is 1.
Altogether, Black has made an even number of moves, since an even number of his/her pieces have made an odd number of moves.
However, White has made an odd number of moves.
Naturally, in this scenario, it must be Black to move. White and Black have clearly made a different number of moves. Thus, it cannot be White to move. Therefore, it is Black to move.
Since it is Black to move, it can be concluded that the answer is definitely 2.
Now stop saying it's 30.
EDIT:
Original problem wanted the product of the x and y coordinates of the move that will give checkmate, where bottom left corner is (0,0).