Christmas Streak 44/88: Halfway Through!
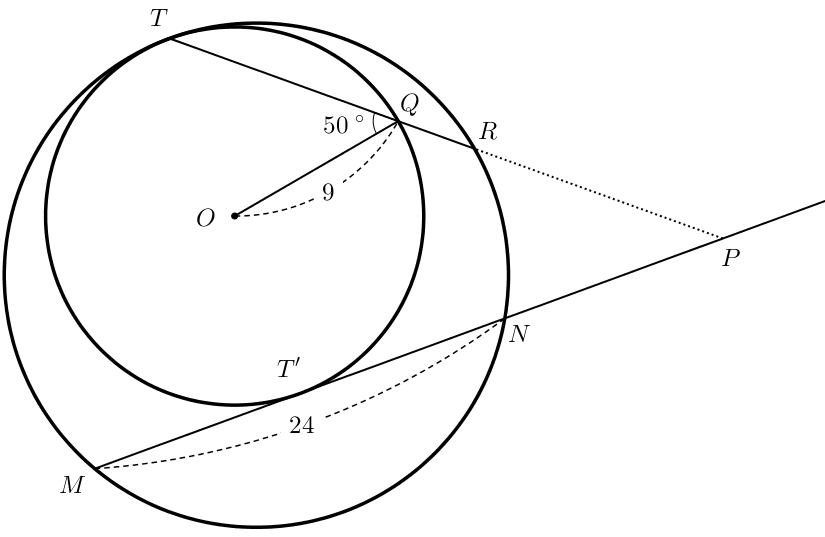
In the diagram, is the midpoint of
Find the value of to 4 decimal places.
This problem is a part of <Christmas Streak 2017> series .
The answer is 2.2500.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Look at this beautiful diagram that will guide you to the answer right away.
T ′ S × T T ′ = M T ′ 2 ⇔ T ′ S = 8 .
It's pretty obvious that ∠ T ′ Q T = ∠ S R T = 9 0 ∘ .
So T ′ Q / / S R .
Therefore, by similarity, Q T : Q R = T T ′ : T ′ S = 1 8 : 8 = 4 9 = 2 . 2 5 0 0 .