Circle Conundrum

Geometry Level 1

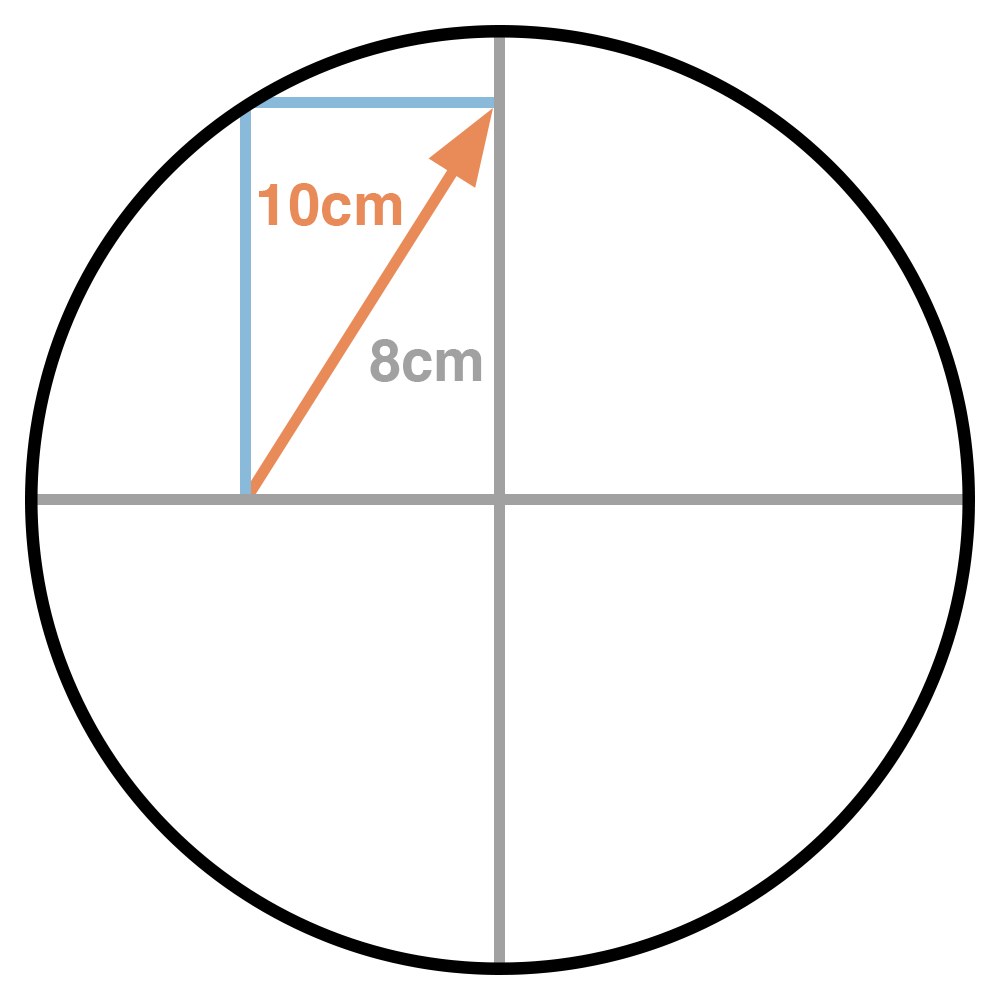
If the area of the circle can be expressed as $A \pi \text{ cm}^2$ , find the value of $A$ .

A rectangle is drawn in a circle so that one vertex lies on the center point of the circle, and the opposite corner lies on the circumference. The height of the rectangle is $8\text{ cm}$ , and its diagonal is $10\text{ cm}$ .

The answer is 100.

1 solution

Fin Moorhouse
Apr 8, 2016

Just flip the diagonal: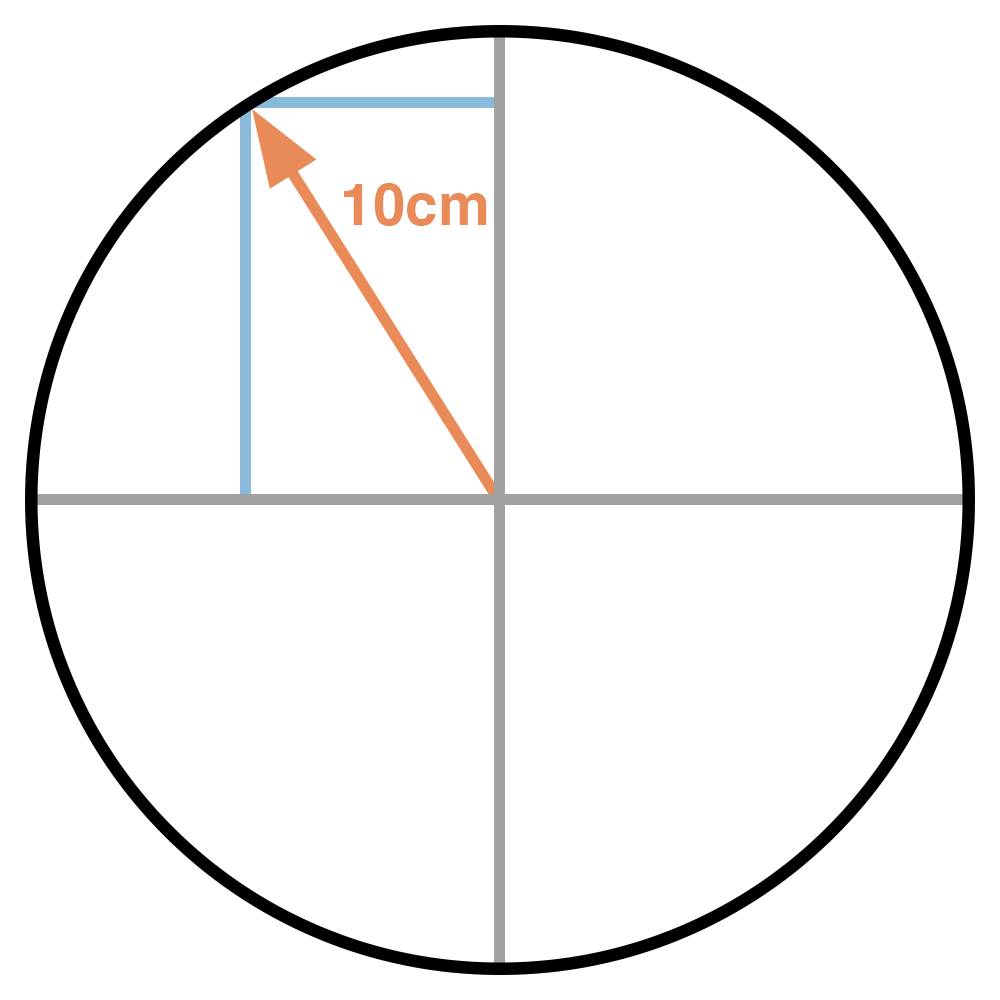 It becomes clear that the radius is $10cm$ , so the area of the circle is $100\pi cm^2$ .

You asked to the nearest integer. Doesn't this imply that you're asking for 100 pi, which is about equal to 314?

John Taylor - 5 years, 2 months ago

Thanks. We have updated the problem statement.

In future, if you spot any errors with a problem, you can “report” it by selecting "report problem" in the “line line line” menu in the top right corner. This will notify the problem creator who can fix the issues.

Brilliant Mathematics Staff - 5 years, 2 months ago

Cheers for this.

Fin Moorhouse - 5 years, 2 months ago

good question John Taylor

Takis Psaltis - 5 years, 1 month ago

Oh!! Great question!

A Former Brilliant Member - 5 years, 2 months ago

Lets apply the Pythagoras theorem. 10^2 = 8^2 + x^2. Therefore part of radius i.e x =6. Now draw a dotted line which forms a quadrilateral with opposite sides equal in length i.e 8cm and 6cm. So the remaining part of radius is also 6cm so total is 12. Therefore answer should be 144πcm^2.

Karan Jain - 5 years, 1 month ago

Sorry, that's wrong. The radius is 10cm.

Fin Moorhouse - 5 years ago

Circle Conundrum

The answer is 100.

1 solution

0 pending reports