Circles

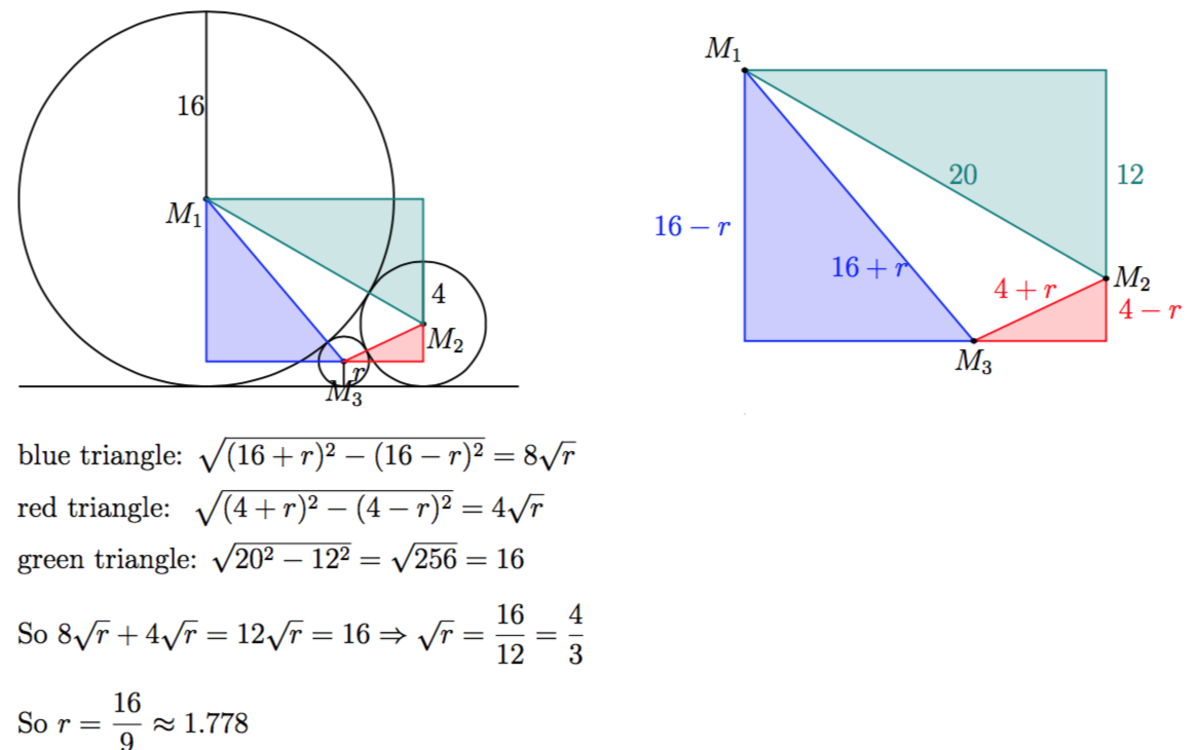
to solve sums like this ... i.e., involving a common tangent ...... there is a formula which can b applied for this case

"1/root(R) = 1/root(R1) + 1/root(R2)"

where R = Radius of small circle; R1 and R2 are the radii of the other two circles

Applying the formula, v get Reqd. radius = 1.77 / 1.78

i wud post the proof of the formula within a few days ...

A specialized case of Descartes' Theorem for three tangential circles and a line is $k_4 = k_1 + k_2 + 2\sqrt{k_1k_2}$ , where $k_1 = \frac{1}{r_1}$ , $k_2 = \frac{1}{r_2}$ , and $k_4 = \frac{1}{r_4}$ . In this problem, $k_1 = \frac{1}{16}$ , $k_2 = \frac{1}{4}$ , and $k_4 = \frac{1}{R}$ . Substituting, we get $\frac{1}{R} = \frac{1}{16} + \frac{1}{4} + 2\sqrt{\frac{1}{16}\frac{1}{4}}$ , and solving we get $R = \frac{16}{9} \approx \boxed{1.77}$