Inscribed Isosceles Triangle

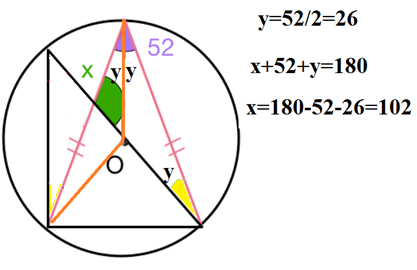
Method 1 : Apply Thales Theorem .

Let's denote the points $A,B,C,D$ and $E$ as shown below.

Because $AOC$ is a straight line that passes through the center $O$ , then the triangle $CBA$ is a right triangle with $\angle CBA = 90^\circ$ by Thales' Theorem.

And note that the triangle $BDA$ is an isosceles triangle with $BD = AD$ , so $\angle DBA = \angle DAB = \dfrac{180^\circ -52^\circ}2 = 64^\circ$ .

Thus $\angle CBE = \angle CBA - \angle DBA = 90^\circ - 64^\circ =26^\circ$ .

Apply the properties of Inscribed Angle Theorem , we have $\angle BCA = \angle BDA = 52^\circ$ .

Because $\angle CEB = \angle DEA = x^\circ$ , and we know the sum of angles of a triangle is $180^\circ$ , so

$\begin{aligned} \angle CBE + \angle BEC + \angle ECB &=& 180^\circ \\ 26^\circ + x^\circ + 52^\circ &=& 180^\circ \\ x^\circ &=& 180^\circ - 26^\circ - 52^\circ \\ x^\circ &=& 102^\circ \\ x &=& \boxed{102} \end{aligned}$

---

Method 2 : Apply Inscribed Angle Theorem only.

Let's denote the points $A,B,C,D$ and $E$ as shown below.

By inscribed angle theorem, $\angle BOA = 2\angle BDA = 2 (52^\circ) = 104^\circ$ .

Because $BO = OA$ represents the radius of the circle, then the triangle $BOA$ is an iscosceles triangle with $\angle OBA = \angle BAO = \dfrac{180^\circ - 104^\circ}2 = 38^\circ$ .

Similarly, because $BD = Ad$ , then the triangle $BDA$ is an isosceles triangle with $\angle BAD = \angle DBA = \dfrac{180^\circ - 52^\circ}2 = 64^\circ$ .

And so, $\angle DAE = \angle DAB - \angle OAB = 64^\circ - 38^\circ = 26^\circ$ .

By referring to triangle $DEA$ , we have

$\begin{aligned} \angle DEA + \angle EAD + \angle ADE &=& 180^\circ \\ x^\circ + 26^\circ + 52^\circ &=& 180^\circ \\ x^\circ &=& 180^\circ - 26^\circ - 52^\circ \\ x^\circ &=& 102^\circ \\ x &=& \boxed{102} \end{aligned}$