Circles and squares
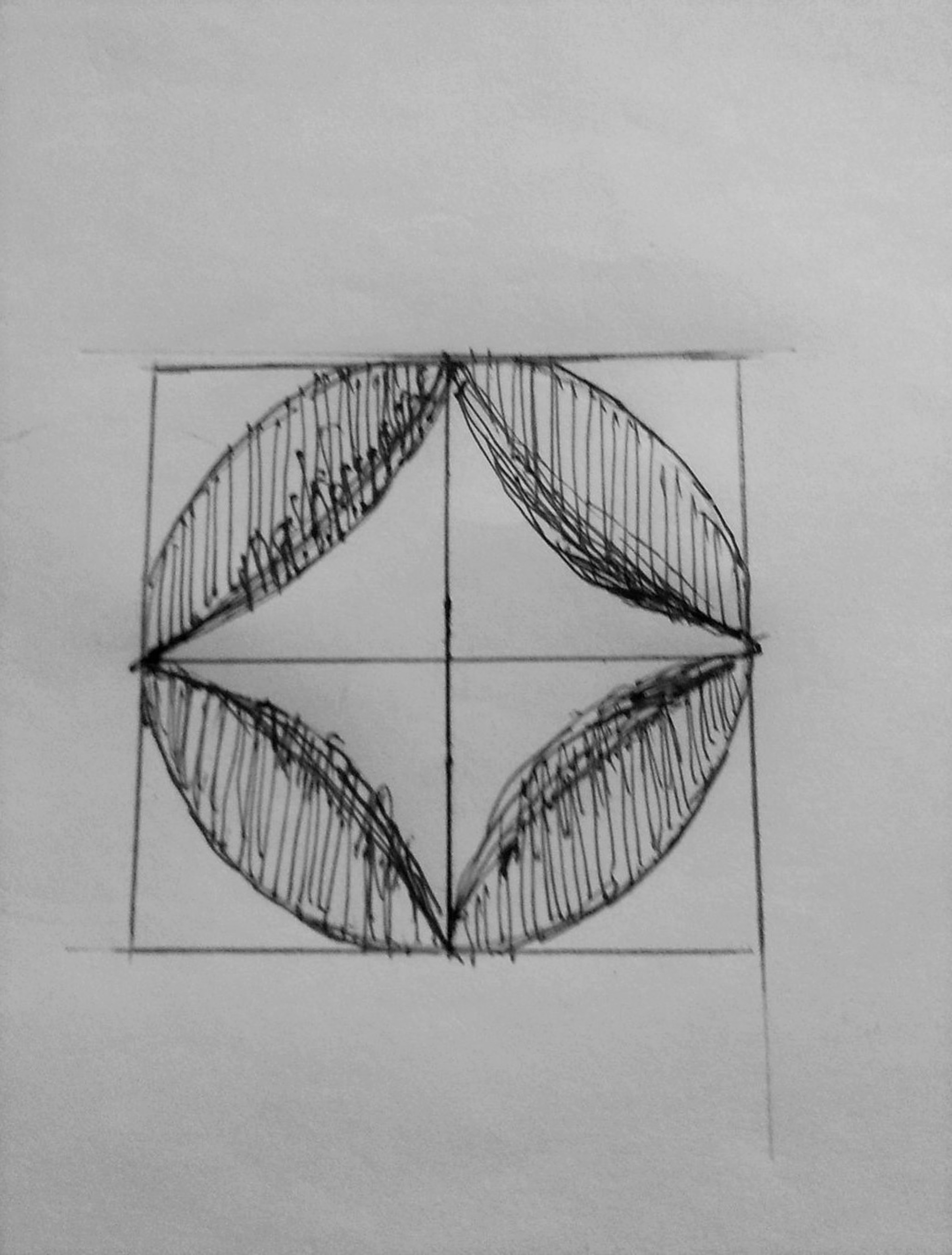
The radius of the circle is . It is inscribed in a square of side . The square, as well as circle, are divided into four equal portions by a horizontal and vertical line. Now arc in each smaller square was converted to four petals (shaded area) by taking a mirror image of them as shown in the diagram. What is the percent of the area of the petals to the total are of the big square?
Give your answer to the nearest integer.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Here each of the small squares has an area (R^2). The area covered by circle in each square is [(pi)R^2]/4 So the empty area in the corner of the small square = (R^2) - [(pi)R^2]/4 = 0.215(R^2) In each square there are two such empty spaces, above and below the petal; so their total area = 0.43(R^2) So area of petal in each square = (R^2)- 0.43(R^2) = 0.57(R^2) So percent area of petal per small square or all the petals together in the big square(they are the same) = [0.57(R^2)/(R^2)] x 100 = 57%