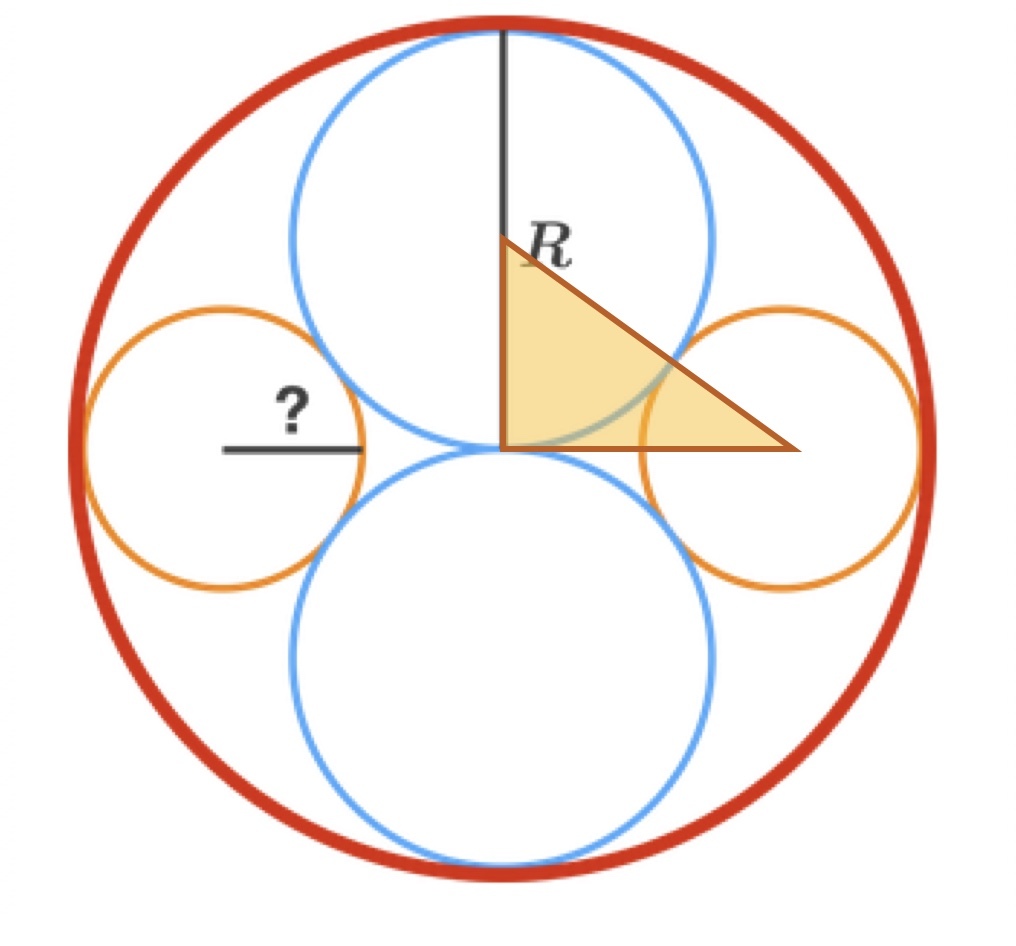
Circular Double Twins

Let the radius of the red circle be $R_1$ and the radius of the orange circle be $R_2$

Then we can find in the figure

• $O_3O_1=\frac{R_1}{2}+R_2$

• $O_3C=R_1-R_2$

• $O_1C=\frac{R_1}{2}$

and we also see that $O_3C$ is tangent of both the blue circles. So $\angle O_1CO_3=90$ .

Therefore we can apply Pythagorean theorum for the right $\Delta O_1CO_3$

$\Rightarrow (R_1-R_2)^2+(\frac{R_1}{2})^2=(\frac{R_1}{2}+R_2)^2$

$\Rightarrow R_1^2+R_2^2-2R_1R_2+\frac{R_1^2}{4}=\frac{R_1^2}{4}+R_2^2+R_1R_2$

$\Rightarrow R_1^2=3R_1R_2$

$\Rightarrow R_2=\boxed{\frac{R_1}{3}}$

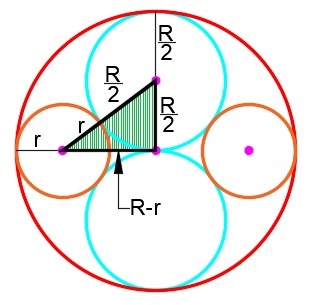
Let $r$ be the unknown radius. The right triangle drawn here has horizontal leg $R - r$ , vertical leg $\tfrac12R$ , and hypotenuse $\tfrac12R + r$ . Pythagoras says $(R - r)^2 + (\tfrac12R)^2 = (\tfrac12R + r)^2.$ $R^2 - 2Rr + r^2 + \tfrac14R^2 = \tfrac14R^2 + Rr + r^2.$ Cancel the $r^2$ , combine like terms, and divide by $R$ to find $\boxed{r = \tfrac13R}.$

The large circle has a radius of $R$ and the medium circles each have a radius of $\frac{R}{2}$ . Then by Descartes' Theorem , $k_4 = k_1 + k_2 + k_3 \pm \sqrt{k_1k_2 + k_2k_3 + k_1k_3}$ where $k_1 = -\frac{1}{R}$ (the reciprocal of the radius of the large circle that is internally tangent to the others), $k_2 = \frac{2}{R}$ (the reciprocal of the radius of the medium circle that is externally tangent to the others), and $k_3 = \frac{2}{R}$ (the reciprocal of the radius of the medium circle that is externally tangent to the others).
Therefore, $k_4 = -\frac{1}{R} + \frac{2}{R} + \frac{2}{R} \pm \sqrt{-\frac{1}{R}\frac{2}{R} + \frac{2}{R}\frac{2}{R} - \frac{1}{R}\frac{2}{R}} = \frac{3}{R}$ , which makes the radius of the fourth smaller circle the reciprocal of $k_4$ or $\boxed{\frac{R}{3}}$ .

$\dfrac{R}{2}=radius~of~the~blue~circle$ and $r=radius~of~the~orange~circle$

Apply pythagorean theorem on the black right triangle,

$\left(\dfrac{R}{2}+r\right)^2=\left(\dfrac{R}{2}\right)^2+(R-r)^2$

$\dfrac{R^2}{4}+2\left(\dfrac{R}{2}\right)(r)+r^2=\dfrac{R^2}{4}+R^2-2Rr+r^2$

$\dfrac{R^2}{4}+Rr+r^2=\dfrac{R^2}{4}+R^2-2Rr+r^2$

$3Rr=R^2$

$\boxed{r=\dfrac{R}{3}}$

Create a triangle with sides: R - r, R/2 and R/2 + r and use Pythagoras. Little r stands for the radius of the smaller circle.

A very simple solution is rotating line R counterclockwise 90 degrees and seeing that the short line is 1/3 the radius

We note that the diameters of the blue circles and the extensions of the diameters of the orange circles meet at the center $O$ of the big red circle. Let the radius of the orange circle be $r$ . Since the radii $\dfrac R2$ and $r$ from the centers $A$ and $C$ of the top blue and the left orange circles respectively are perpendicular to their common tangent, $AC = \dfrac R2 + r$ is a straight line. Now we have:

$\begin{aligned} BC + \color{#3D99F6}CO & = BO & \small \color{#3D99F6} \text{By Pythagorean theorem} \\ r + \color{#3D99F6}\sqrt{AC^2-AO^2} & = R \\ r + \sqrt{\left(\frac R2 + r\right)^2-\left(\frac R2\right)^2} & = R \\ \sqrt{\left(\frac R2 + r\right)^2-\left(\frac R2\right)^2} & = R - r & \small \color{#3D99F6} \text{Squaring both sides} \\ \left(\frac R2 + r\right)^2-\left(\frac R2\right)^2 & = R^2 - 2Rr + r^2 \\ \frac {R^2}4 + Rr + r^2 - \frac {R^2}4 & = R^2 - 2Rr + r^2 \\ 3Rr & = R^2 \\ \implies r & = \boxed{\dfrac R3} \end{aligned}$

Well, everyobdy here have drawn the triangle, so watch it and read this. By using the hint: $\frac{R^2}{4}+(R-x)^{2}=(\frac{R}{2}+x)^2$ $\Rightarrow \frac{R^2}{4}=(\frac{R}{2}+x+R-x)(\frac{R}{2}+x-R+x)$ $\Rightarrow \frac{R}{6}=2x-\frac{R}{2} \Rightarrow x=\frac{R}{3}$

Solve this equation.

(R-x)² +(R/2)²= (X+(r/2))².

Where R is as given in question and x as the radius of smaller circle.

(Join the centres of all the circles. And then observe the right triangle formed)

(1st point- centre of largest circle

2nd point- centre of medium sized
Circle

3rd point- centre of smallest circle)

*All the above points are a part of the Rhombus formed in step 1

On solving the equation We get 4R² =12Rx On solving further x=R/3