Circus dog
A small circus dog walks on a rolling solid cylinder. The dog remains at a fixed height above the ground corresponding to the angle
θ
=
3
0
∘
. If the mass of the cylinder is 4 times larger than the mass of the dog, find the acceleration
a
of the cylinder's center of mass
in m/s
2
. Assume that the cylinder rolls without slipping.
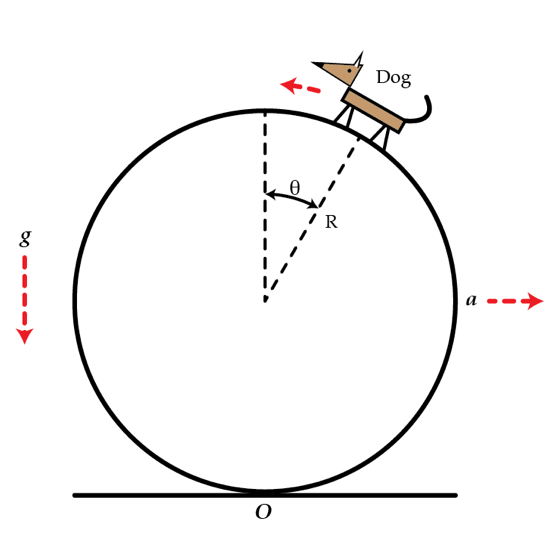
Details and assumptions
- The acceleration of gravity is − 9 . 8 m/s 2 .
The answer is 0.62.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
6 solutions
You are missing another parenthesis after cos(pi/6)
Consider the forces on the dog (mass m): normal (N), friction (f1) and weight. Orienting the axes to coincide with the direction of acceleration gives:
m g = N c o s θ + f 1 s i n θ [equation A]
m a = N s i n θ − f 1 c o s θ [B]
Consider the dog and the cylinder as one object. The external forces on this object are the friction (f2) and the normal force from the floor, and the total weight. We have:
f 2 = 5 m a [C]
Consider the torques on the cylinder (radius R). Only frictional forces provide torque. Noting that, a = R alpha (no slipping), and that the moment of inertia of a uniform solid cylinder (mass m, radius R) about its central axis is given by ( 1 / 2 ) m R 2 , we have:
f 1 R − f 2 R = ( 1 / 2 ) ( 4 m ) ( R a ) [D]
Simplifying the previous equation:
f 1 − f 2 = 2 m a [E]
From the previous equation and C:
f 1 = 7 m a [F]
Using C and F on B, and that θ = 3 0 degrees, N can be solved in terms of ma. Substituting N and f1 in A, will allow us to cancel m, and express a in terms of g:
a = g / ( 1 4 + 3 )
We can put all the forces that act on the sphere, we'll use the Cartesian system:
x.i -> parallel to the floor y.j -> perpendicular to the floor
Now we can write the forces: We can use the draw of the problem to see the vectors
1) for the sphere
Friction force between the sphere and the floor (Fat_1)
Fat 1 = Fat 1.i
Friction force between the sphere and the dog
Fat 2 = Fat 2.cos(30).i - Fat_2.sin(30).j
Gravitational force
P = -4mg.j
Contact force between the sphere and the dog
N 2 = -N 2.sin(30) - N_2.cos(30)
The contact force between the sphere and the floor
N 1 = N 1.j
2) for the dog
The contact force between the dog and the sphere, from the third newton's law we get that the modulus of the forces are the same
N 2 = N 2.sin(30).i + N_2.cos(30).j
Gravitational force
p = -mg.j
Friction force between the sphere and the dog, from the third newton's law we get that the modulus of the forces are the same
Fat 2 = -Fat 2.cos(30).i + Fat_2.sin(30).j
Now we have to analize the resulting torque acting on the sphere:
Since the sphere is rotating with a w on the z direction of our referencial, we can conclude that
Torque = I.α = (1/2).α.M.R^2 = 2.α.mR^2 = (Fat 2 - Fat 1).R Where R is the cilinder's radius, α is the angular acceleration and I is the inertia momentum
But there is no slipping so we can say that v = wR -> a = αR Where a is the cilinder's center of mass' acceleration
So we have that
Fat 1 = Fat 2 - 2ma (eq.01)
Now we can apply the Newton's second law
For the sphere:
On the x axis
Fat 1 + Fat 2.cos(30) - N_2.sin(30) = 4ma
Substituting Fat_1 from the (eq.01) we have that
Fat 2 = (N 2 + 12ma)/(sqrt(3) + 2) (eq.02)
Now we analize the dog
In the x axis
N 2.sin(30) - Fat 2.cos(30) = ma
Fat 2 = N 2(sqrt(3)/3) - 2ma(sqrt(3)/3) (eq.03)
Substituting Fat_2 from the (eq.02) on (eq.03) we get that
N_2 = ma(7.sqrt(3) + 2) (eq.04)
Now on the y axis
Fat 2.sin(30) + N 2.cos(30) = mg
Substituting Fat_2 we get that
N_2 = (mg.sqrt(3) + ma)/2
Now we subistitute N_2 from the (eq.04)
We get that
a = g/(14 + sqrt(3)) = 0.62 m/s^2
the dog must be having just the horizontal acceleration a as same as the cylinder and also 0 vertical acceleration. Thus taking normal force acting between dog and cylinder as N and corresponding friction as f1 , also friction force from ground on cylinder as f2 resp. we get by writing force eqn. for dog in horizontal and vertical direction and on cylinder in horizontal direction resp. Nsin30-f1cos30=ma.....(1) Ncos30+f1sin30=mg.....(2) f1cos30+f2-Nsin30=4ma...(3) Also writing torque eqn. and applying condn. for pure rolling, (f1-f2)=4ma/2......(4) thus solving above eqns. a=0.62m/s^2
This problem can be easily solved by looking at the dog-cylinder system as a whole. The only external torque with respect to the point O is τ = m g R sin θ where m is the mass of the dog and R the radius of the cylinder. Now, the angular momentum with respect to point O can be written as L = L c y l i n d e r + L d o g The angular momentum of the cylinder with respect to the point O is just L c y l i n d e r = I 0 ω = ( 2 M R 2 + M R 2 ) ω = 2 3 M R 2 ω where M is the mass of the cylinder. Here, we made use of the Parallel Axis Theorem. The angular momentum of the dog is L d o g = m v R ( 1 + cos θ ) . Since the the cylinder rolls without slipping we have that v = ω R . Thus, we obtain the total angular momentum of the dog-cylinder system L = ( 2 3 M + m ( 1 + cos θ ) ) R v Since τ = d t d L and a = d t d v we find that a = 2 3 M + m ( 1 + cos θ ) m g sin θ = 7 + cos θ g sin θ = 0 . 6 2 m/s 2 .
the dog must be having just the horizontal acceleration a as same as the cylinder and also 0 vertical acceleration. Thus taking normal force acting between dog and cylinder as N and corresponding friction as f1 , also friction force from ground on cylinder as f2 resp. we get by writing force eqn. for dog in horizontal and vertical direction and on cylinder in horizontal direction resp. Nsin30-f1cos30=ma.....(1) Ncos30+f1sin30=mg.....(2) f1cos30+f2-Nsin30=4ma...(3) Also writing torque eqn. and applying condn. for pure rolling, (f1-f2)=4ma/2......(4) thus solving above eqns. a=0.62m/s^2
As the cylinder rolls without slipping, it is convenient to place the instantaneous axis of rotation at the point of contact between the ground and the cylinder.
According to the definition, τ = d L / d t = d L c y l / d t + d L d o g / d t
Let the mass of dog be m and that of cylinder is 4 m . We have L c y l = 6 m R 2 ω = 6 m R v and similarly L d o g = m v R ( 1 + cos ( π / 6 ) ) (The dog performs only horizontal motion and v represents the linear velocity.)
The time derivatives of angular momenta can be easily calculated. The only torque about the axis is due to the weight of the dog i.e. m g R sin ( π / 6 ) .
Hence, m g R sin ( π / 6 ) = 6 m R a + m a R ( 1 + cos ( π / 6 )
⇒ a = 7 + cos ( π / 6 ) g sin ( π / 6 ) ⇒ a = 0 . 6 2 3 m / s 2