Clark Gable?

The only way the person with highest number could not be between 2/3 and 1 is if all three were less than 2/3.

$\implies P = 1 - (\frac{2}{3})^3 = \dfrac{19}{27}$

$19 + 27 = \boxed{46}$

Let Able's number be $x$ , Mable's be $y$ , and Clark Gable's be $z$ . Then their numbers can be plotted as a point on an $xyz$ -coordinate system inside a unit cube. Divide the unit cube into $27$ congruent $\frac{1}{3} \times \frac{1}{3} \times \frac{1}{3}$ smaller cubes (like a Rubik's cube). Then the following green cubes have at least one coordinate between $\frac{2}{3}$ and $1$ , and the following red cubes do not.

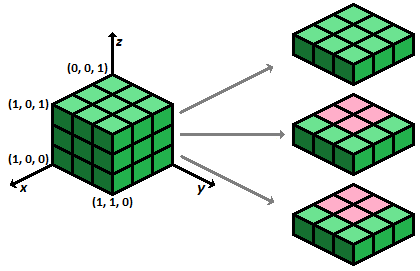
Since $19$ out of $27$ cubes are green, the probability is $\frac{19}{27}$ , so $a = 19$ , $b = 27$ , and $a + b = \boxed{46}$ .

Lectures for students NSU

$\int_{\frac{2}{3}}^1 3 x^2 dx=\frac{19}{27}$