Close approximation

Geometry Level 3

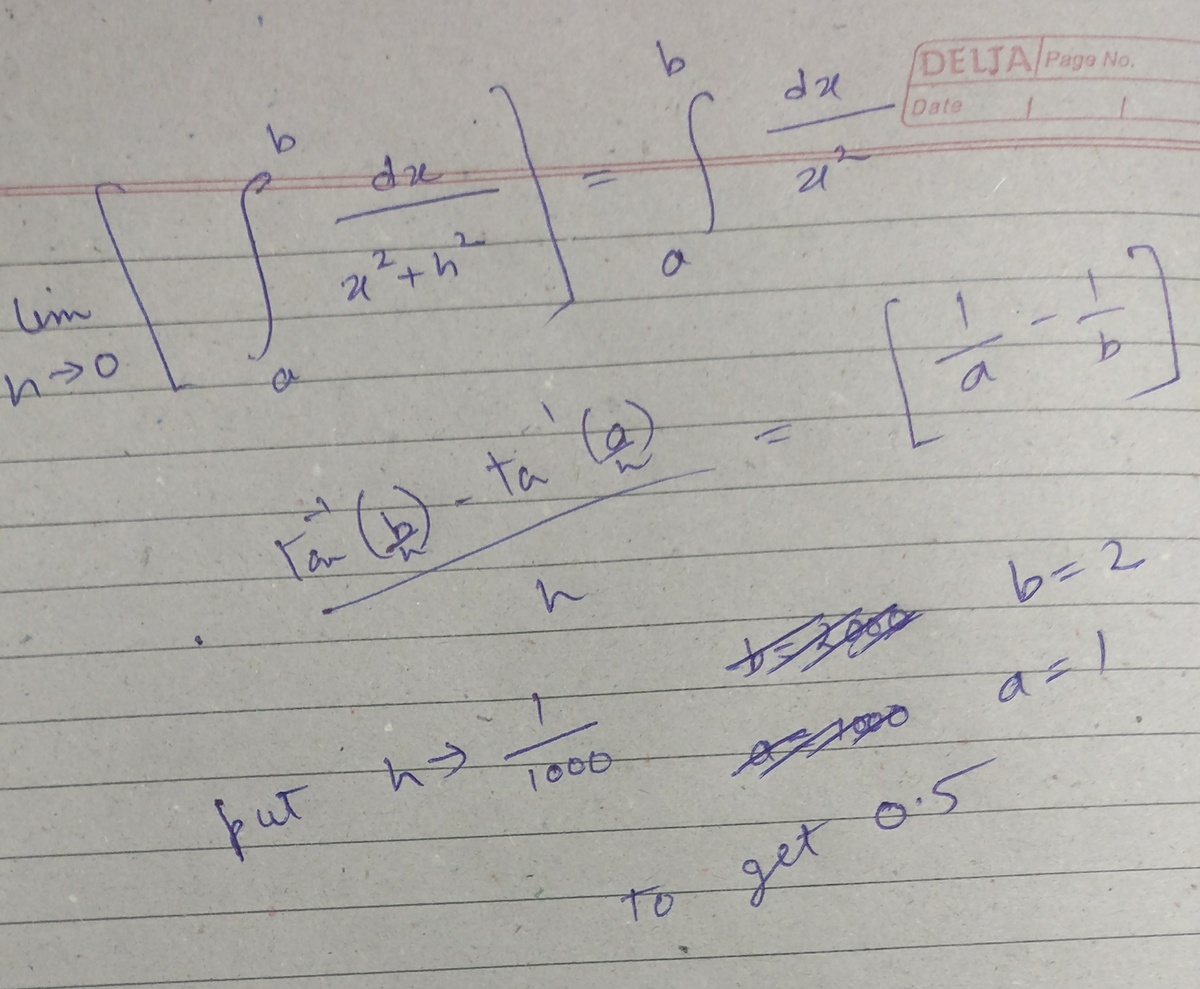
( tan ⁡ − 1 ( 2000 ) − tan ⁡ − 1 ( 1000 ) ) × 1000 = ? \large \left(\tan^{-1}(2000)-\tan^{-1}(1000)\right)\times1000 = \, ?

Round your answer to 3 decimal places.


The answer is 0.5.

Subh Mandal by subh mandal , 22, India

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

2 solutions

Anirudh Sreekumar
Mar 23, 2017

tan ⁡ − 1 ( 2000 ) − tan ⁡ − 1 ( 1000 ) = tan ⁡ − 1 ( 2000 − 1000 1 + 2000 × 1000 ) = tan ⁡ − 1 ( 1000 1 + 2000 × 1000 ) \begin{aligned} \tan^{-1}(2000)-\tan^{-1}(1000)&=\tan^{-1}\left(\dfrac{2000-1000}{1+2000\times1000}\right)\\&=\tan^{-1}\left(\dfrac{1000}{1+2000\times1000}\right)\end{aligned}

we can see that the angle, θ = tan ⁡ − 1 ( 1000 1 + 2000 × 1000 ) \theta=\tan^{-1}\left(\dfrac{1000}{1+2000\times1000}\right) is really small

so,we can use the small angle approximation θ ≈ tan ⁡ θ \theta\approx\tan\theta

1000 θ ≈ 1000 tan ⁡ θ ≈ 1000 × ( 1000 1 + 2000 × 1000 ) ≈ 0.5 \begin{aligned}1000\hspace{1mm} \theta&\approx1000\tan\theta\\&\approx1000\times\left(\dfrac{1000}{1+2000\times1000}\right)\\&\approx0.5\end{aligned}

2 Helpful 0 Interesting 0 Brilliant 0 Confused
Subh Mandal
Mar 22, 2017

2 Helpful 0 Interesting 0 Brilliant 0 Confused

While the limit gives a roadmap for the problem, we would need to do some error analysis to support setting h = 1 1000 h = \frac{1}{1000} to approximate the integral.

In this case, we can use simple estimates to show ∣ ∫ 1 2 d x x 2 − ∫ 1 2 d x x 2 + h 2 ∣ ≤ h 2 \left|\int_1^2 \frac{dx}{x^2} - \int_1^2 \frac{dx}{x^2+h^2}\right| \leq h^2 so the error is at most h 2 = 1 0 − 6 h^2 = 10^{-6} , which is well under the required input accuracy.

Brian Moehring - 4 years, 2 months ago

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...