Co-ordinates of circumcenter?

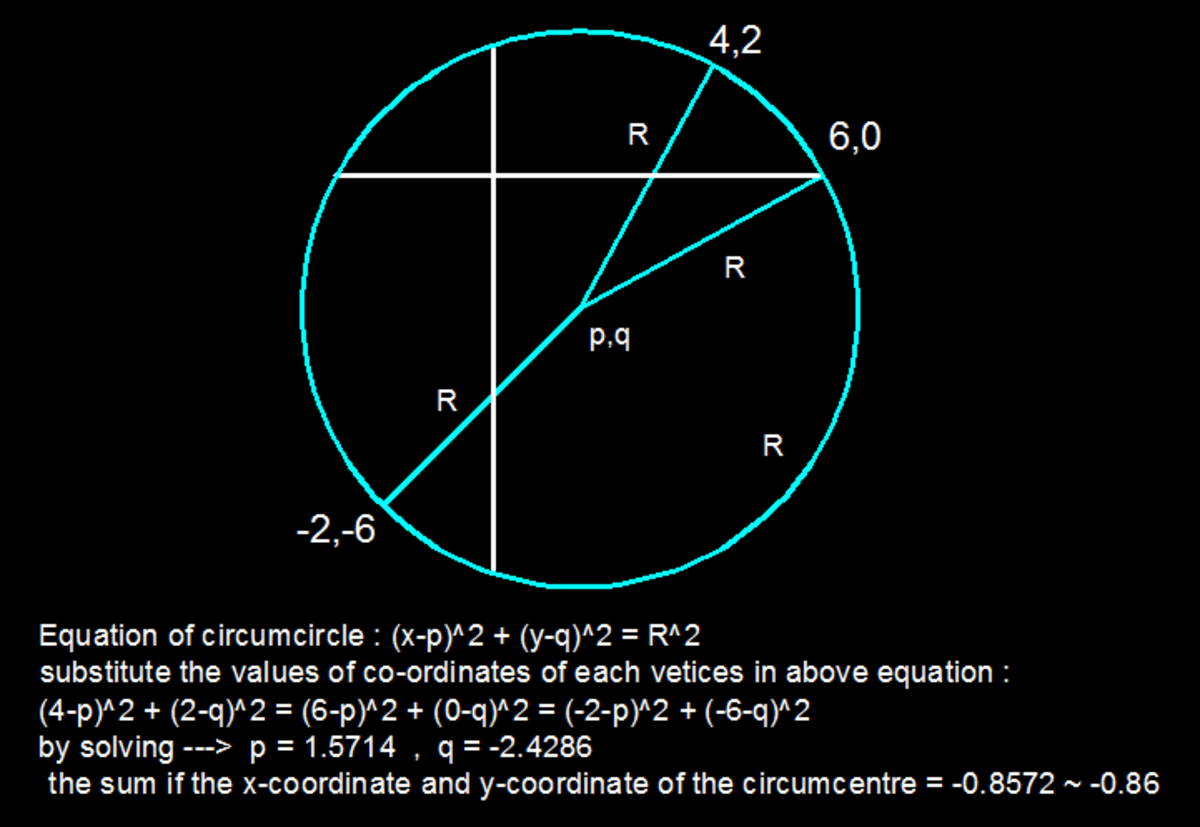
The circumcentre is the point of intersection of the perpendicular bisectors.
Let $(-2,-6)$ be point A, $(4,2)$ be point B and $(6,0)$ be point C.

Midpoint of AC = $\left(\dfrac{-2 + (-6)}{2},\dfrac{-6 + 0}{2}\right) = (2,-3)$

Slope of AC = $\dfrac{0 - (-6)}{6 - (-2)} = \dfrac{3}{4}$
Slope of perpendicular bisector of AC = $\dfrac{-1}{\frac{3}{4}} = -\dfrac{4}{3}$

Equation of perpendicular bisector of AC:-
$m = \dfrac{y - y_1}{x - x_1}\\ \\ -\dfrac{4}{3} = \dfrac{y - (-3)}{x - 2}\\ \\ -4\left(x - 2\right) = 3\left(y + 3\right)\\ 4x + 3y = -1 \longrightarrow \boxed{1}$

Midpoint of AB = $\left(\dfrac{4 + (-2)}{2},\dfrac{2 + (-6)}{2}\right) = (1,-2)$

Slope of AB = $\dfrac{2 - (-6)}{4 - (-2)} = \dfrac{4}{3}$
Slope of perpendicular bisector of AC = $\dfrac{-1}{\frac{4}{3}} = -\dfrac{3}{4}$

Equation of perpendicular bisector of AC:-
$m = \dfrac{y - y_2}{x - x_2}\\ \\ -\dfrac{3}{4} = \dfrac{y - (-2)}{x - 1}\\ \\ -3\left(x - 1\right) = 4\left(y + 2\right)\\ 3x + 4y = -5 \longrightarrow \boxed{2}$

Multiplying $\boxed{1}$ by 3 and $\boxed{2}$ by 4, we get:-
$12x + 9y = -3 \longrightarrow \boxed{3}\\ 12x +16y = -20 \longrightarrow \boxed{4}$

Subtracting $\boxed{4}$ from $\boxed{3}$ , we get:-
$-7y = 17\\ y = -\dfrac{17}{7}\\ y = -2.42$

Substituting $y = -\dfrac{17}{7}$ in $\boxed{3}$ , we get:-
$12x + 9×-\dfrac{17}{7} = -3\\ \\ 12x - \dfrac{153}{7} = - 3\\ \\ 12x = -3 + \dfrac{153}{7}\\ \\ 12x = \dfrac{-21 + 153}{7}\\ \\ 12x = \dfrac{132}{7}\\ \\ x = \dfrac{132}{14}\\ \\ x = 1.57$

So, co-ordinates of circumcentre = $(x,y) = (1.57,-2.42)$
So, $x + y = 1.57 + (-2.42) = \color{#3D99F6}{\boxed{-0.86}}$