warm up problem 15: concentric circles easy.
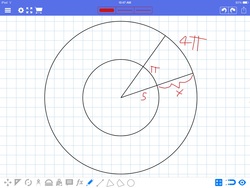 The arc lengths of the small and large circles is
π
and
4
π
respectively.
The arc lengths of the small and large circles is
π
and
4
π
respectively.
If the radius of the smaller circle is 5 , find the length of the segment labeled x .
The answer is 15.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
As per theorem , angle = arc/radius . so angle is same .then compare both angle .
(x/5)=(4x/(x+5)) x=15
By ratio and proportion, we have
π 5 = 4 π 5 + x ⟹ π 2 0 π = 5 + x ⟹ 2 0 − 5 = x ⟹ x = 1 5
Since all circles are similar, we can set up a proportion:
5 π = x + 5 4 π
π x + 5 π = 2 0 π
x = 1 5