Colliding charged plates !
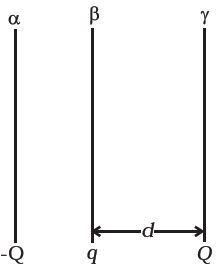 Two fixed, identical conducting plates
&
, each of surface area
are charged to
and
, respectively, where
. A third identical plate (
), free to move is located on the other side of the plate with charge
at a distance
. The third plate is released and collides with the plate
. Assume the collision is elastic and the time of collision is sufficient to redistribute charge amongst
&
.
Two fixed, identical conducting plates
&
, each of surface area
are charged to
and
, respectively, where
. A third identical plate (
), free to move is located on the other side of the plate with charge
at a distance
. The third plate is released and collides with the plate
. Assume the collision is elastic and the time of collision is sufficient to redistribute charge amongst
&
.
Find the velocity of the plate after the collision and at a distance from the plate .
If
then, find .
The answer is 5.000.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
First there is a net force on third plate
F = 2 ϵ 0 S Q ( Q − q )
Now you can find the speed of plate in the moment of the collision.
v 1 2 = 2 ϵ 0 m Q ( Q − q ) d
So now there is a key part of the problem during the collision the charge will redistrubate over the second and third plates. Let q 1 be the new charge of second and q 2 the new charge of the third plate. While they are connected net eletric field between them is 0.
= > − Q + q 1 − q 2 = 0
And we know also that:
q + Q = q 1 + q 2
= > q 1 = Q + q / 2 and q 2 = q / 2
Now if you do the same thing with forces from the beginning you will easy find:
v f = ( Q − q / 2 ) 2 ϵ 0 m S d