Common part volume of six crossing cylinders
The solid common to 2 (or 3) right circular cylinders of equal radii intersecting at right angles is called the Steinmetz solid .
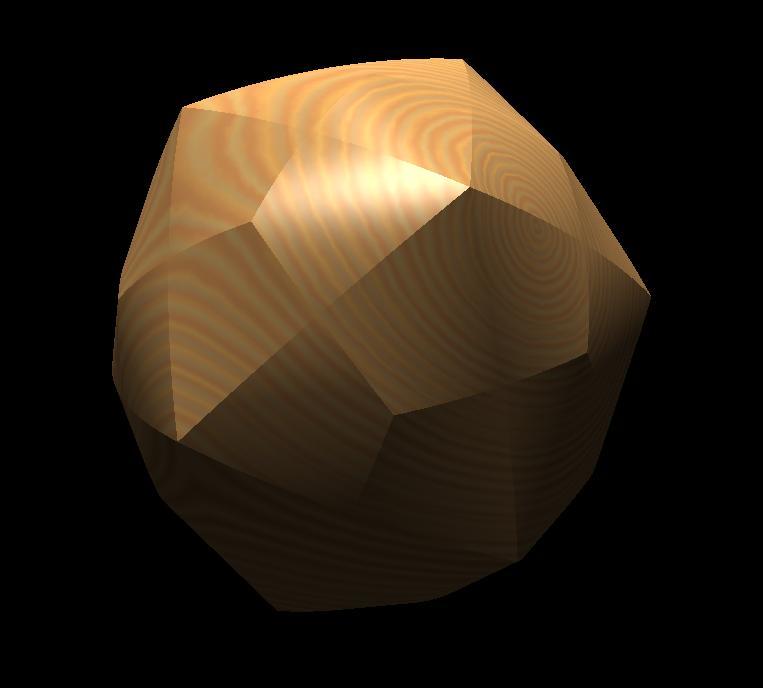
Find the volume of the figure common to 6 crossing cylinders with radius orthogonal to the faces of the dodecahedron (thus, this time, not intersecting at right angles).
If the answer can be expressed as where are positive integers and are square-free, give the value of
For the picture, go to http://www.povray.org/ .
The answer is 55.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Outline:
Relation between volume and surface area . Note that the boundary of our solid is restricted by cylinders, each of which has the same radius R = 1 and all of which have their axes pass through the solid's centre. Consider a cylinder and take a ring-shaped part of its surface area A = 2 π r h . Also consider the volume that is enclosed between the surface area and the centre of the solid, by connecting each point of the area boundary by a straight line to the centre. The enclosed volume is V = ∫ 0 R 2 π r ⋅ h R r d r = 3 2 π R 2 h = 3 1 R A . Using standard arguments, this can easily be generalised to any section of cylindrical area. Because we have R = 1 , for our purposes we can use V = 3 1 A
Axis vectors . I will place a dodecahedron such that is centre is at the origin, its 'top' face is parallel to the x y -plane, having a vertex on the first quadrant of the x z -plane, i.e. y = 0 , x > 0 , z > 0 .
Now unit normal vectors to the six uppermost planes are n 0 = ( 0 , 0 , 1 ) and n i = ( 5 2 cos α i , 5 2 sin α i , 5 1 ) for i=1, 2, 3, 4, 5 Here α 1 = π / 5 , α 2 = 3 π / 5 , α 3 = π , α 4 = 7 π / 5 , α 5 = 9 π / 5 . Check that ∣ n i ∣ = 1 and n 0 ⋅ n 1 = n 1 ⋅ n 2 etc.
Identify a cylindrical area Note that the vertical cylinder x 2 + y 2 = 1 defines the surface of the solid at the point p = ( 1 , 0 , 0 ) , because its axis ( 0 , 0 , 1 ) is at right angles to p . We are looking for the (boundaries of) the area that is defined by this cylinder.
For any point p on the vertical cylinder we can set p = ( cos θ , sin θ , z ) . If also ∣ n i × p ∣ = 1 , it is on the cylinder around axis n i as well. ∣ n i × p ∣ 2 = 5 1 ( 2 sin α ⋅ z − sin θ , cos θ − 2 cos α ⋅ z , 2 cos α sin θ − 2 sin α cos θ ) 2 = 5 1 ( 4 z 2 − 4 cos ( θ − α ) + 1 + 4 sin 2 ( θ − α ) = 1 which solves to z = 2 cos ( θ − α ) ± 5 cos ( θ − α ) so that every value of θ generates two points on the intersection of the two cylinders: p 1 = ( cos θ , sin θ , φ cos ( θ − α ) ) , p 2 = ( cos θ , sin θ , − φ 1 cos ( θ − α ) ) where φ = 2 1 + 5 .
Note that p 1 and p 2 coincide when θ − α = ± 2 π (mod π), so these values for θ reflect an intersection that is on the solid's surface. Fig. 1a. Top view, as seen from the positive z-axis
Fig. 1a. Top view, as seen from the positive z-axis
Fig. 1 Free-hand sketches of a dodecahedron that is circumscribed about the unit sphere. Colours show which cylinder will locally define the resulting solid. Axes of the cylinders are marked by dots or arrows in corresponding colours.
It turns out that the area containing point ( 1 , 0 , 0 ) is bordered by the intersection of cylinder 0 with cylinder 2 for positive y and by the intersection of cylinder 0 with cylinder 4 for negative y. [Cylinders numbered according to their axis vector indices.]
Calculate area and volume For cylinder 2, the upper boundary (where p 1 and p 2 coincide) is α + 2 π (mod π) = 1 0 π . The following integral gives half the area: A h a l f s e c t i o n = ∫ 0 π / 1 0 ( φ + φ − 1 ) ∣ cos ( θ − α 2 ) ∣ d θ = 5 ∫ − 3 π / 5 − π / 2 ∣ cos u ∣ d u = 5 ( 1 − sin ( 3 π / 5 ) ) = 5 ( 1 − 8 5 + 5 )
The total area consists of 1 2 × 5 × 2 = 1 2 0 times this area, and hence the volume equals one third of that:
V = 4 0 5 ( 1 − 8 5 + 5 ) = 4 0 5 − 1 0 1 0 5 + 5 ≈ 4 . 3 7 7 6
Sanity check: the volume should be slightly larger than that of a sphere of radius 1, which is 3 4 π 1 3 ≈ 4 . 1 9 .
The answer to submit then is 4 0 + 5 + 1 0 = 5 5