Common Tangent Lines and Areas.
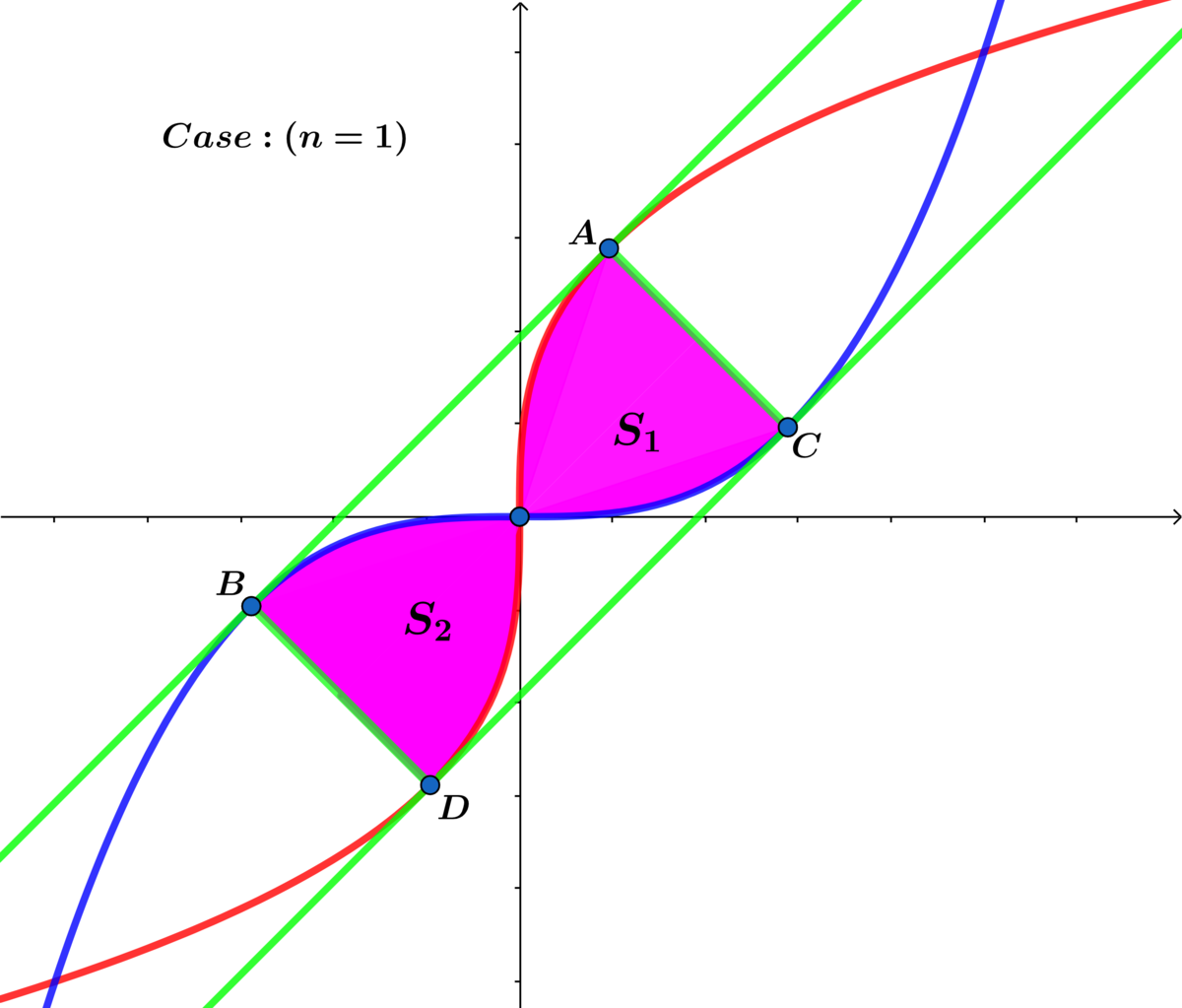
Let be a positive integer, and
and have common tangent lines at points and and and respectively.
Let be the area of the bounded regions and as shown above.
Find .
The answer is 1.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Let f ( x ) = x 2 n + 1 1 and g ( x ) = x 2 n + 1
First we find the area A ( n ) of the regions R 1 and R 2 above.
From the graph above R ( 0 , 0 ) ∘ R y = x ( x 0 , y 0 ) = ( − y 0 , − x 0 )
Using A : ( x 0 , y 0 ) and B : ( − y 0 , − x 0 ) ⟹ slope m A B = 1 ⟹
f ′ ( x 0 ) = 2 n + 1 1 ( x 0 1 ) 2 n + 1 2 n = 1 ⟹ x 0 = ( 2 n + 1 1 ) 2 n 2 n + 1 ⟹
A : ( ( 2 n + 1 1 ) 2 n 2 n + 1 , ( 2 n + 1 1 ) 2 n 1 ) and B : ( − ( 2 n + 1 1 ) 2 n 1 ) , − ( 2 n + 1 1 ) 2 n 2 n + 1 )
⟹ y = x + ( 2 n ) j ( n ) , where j ( n ) = ( 2 n + 1 1 ) 2 n 2 n + 1
⟹ A 1 ( n ) = ∫ 0 j ( n ) ( x + ( 2 n ) j ( n ) − x 2 n + 1 1 ) d x =
2 1 x 2 + ( 2 n ) j ( n ) x + ( 2 n + 2 2 n + 1 ) x 2 n + 1 2 n + 2 ∣ 0 j ( n ) =
( 2 4 n + 1 ) ( j ( n ) ) 2 − ( 2 n + 2 2 n + 1 ) ( j ( n ) ) 2 n + 1 2 n + 2 =
( 2 4 n + 1 ) ( 2 n + 1 1 ) n 2 n + 1 − ( 2 n + 2 2 n + 1 ) ( 2 n + 1 1 ) n n + 1 =
2 1 ( n + 1 n ) ( 2 n + 1 1 ) n 2 n + 1
and
A 2 ( n ) = ∫ − ( 2 n + 1 1 ) 2 n 1 0 ( x + ( 2 n ) j ( n ) − x 2 n + 1 ) d x =
2 1 x 2 + ( 2 n ) j ( n ) x − ( 2 n + 2 1 ) x 2 n + 2 ∣ − ( 2 n + 1 1 ) 2 n 1 0 =
− ( 2 1 ( 2 n + 1 1 ) n 1 − ( 2 n ) ( 2 n + 1 1 ) n n + 1 − ( 2 n + 2 1 ) ( 2 n + 1 1 ) n n + 1 ) =
− ( 2 1 ( 2 n + 1 1 ) n 1 − ( 2 n + 1 1 ) n n + 1 ( 2 ( n + 1 ) ( 2 n + 1 ) 2 ) ) =
2 1 ( n + 1 n ) ( 2 n + 1 1 ) n 1
⟹ A R 1 ( n ) = A 1 ( n ) + A 2 ( n ) = ( n + 1 n ) ( ( 2 n + 1 ) 2 2 n 2 + 2 n + 1 ) ( 2 n + 1 1 ) n 1 = A R 2 ( n )
⟹ lim n → ∞ A R 1 ( n ) = 2 1 lim n → ∞ ( 2 n + 1 1 ) n 1
and
lim n → ∞ ( 2 n + 1 1 ) n 1 = lim n → ∞ e ( n ln ( 2 n + 1 1 ) ) =
e lim n → ∞ ( n ln ( 2 n + 1 1 ) ) = e lim n → ∞ ( 2 n + 1 − 2 ) = e 0 = 1
⟹ lim n → ∞ A R 1 ( n ) = 2 1 ⟹
Total Area A = n → ∞ lim A ( n ) = 2 n → ∞ lim A R 1 ( n ) = 1 .
Next we find the rectangular area A A B D C ( n ) .
Using the coordinates of points A , B , C above and point D : ( − x 0 , − y 0 ) we have:
A C = 2 ∣ y 0 − x 0 ∣ and A B = 2 ∣ y 0 + x 0 ∣ ⟹
A A B D C ( n ) = 2 ∣ y 0 2 − x 0 2 ∣ = 2 ∣ ( 2 n + 1 1 ) n 1 − ( 2 n + 1 1 ) n 2 n + 1 ∣ =
2 ( 2 n + 1 1 ) n 1 ( 1 − ( 2 n + 1 1 ) 2 ) = ( 2 n + 1 ) 2 2 ( 4 n ) ( n + 1 ) ( 2 n + 1 1 ) n 1 .
From above lim n → ∞ ( 2 n + 1 1 ) n 1 = 1 ⟹ A ∗ = n → ∞ lim A A B D C ( n ) = 2
⟹ lim n → ∞ A T ( n ) = A ∗ − A = 1