Comparable Areas

Geometry Level 2

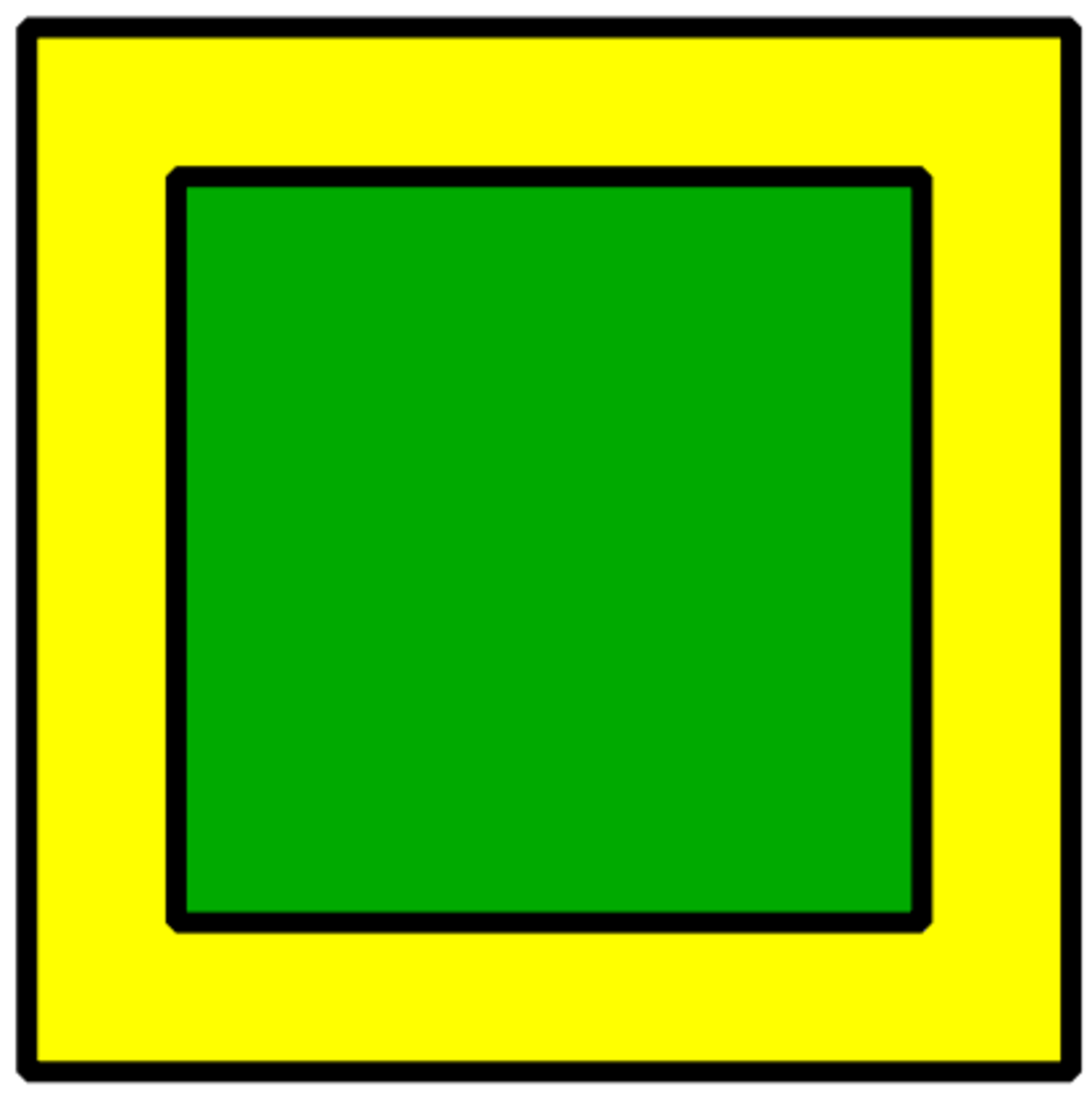
The above shows a square that is inside another square such that the area of the yellow region and the green region are equal.

What is the ratio of the perimeter of the small square and the large square?

\sqrt4 : 4

\sqrt2 : 2

\sqrt3 : 3

\sqrt5 : 5

1 solution

Tom Engelsman
Jan 31, 2021

Let $s$ be the side length of the larger square and $s-2x$ be that of the smaller square. If the yellow and green areas are equal, then we have:

$(s-2x)^2 = s^2 - (s-2x)^2 \Rightarrow 8x^2 - 8sx + s^2 = 0 \Rightarrow x = \frac{8s \pm \sqrt{64s^2-4(8)(s^2)}}{16} \Rightarrow \frac{2 \pm \sqrt{2}}{4} \cdot s$

Since we require $x < s$ we admit only the smaller root, we now compute the ratio of perimeters:

$\frac{P_{small}}{P_{large}} = \frac{4(s-2x)}{4s} = \frac{s - \frac{2 - \sqrt{2}}{2} \cdot s}{s} = \boxed{\frac{\sqrt{2}}{2}}.$

A more direct way is:

The ratio of the areas of the 2 squares is $1: 2$
Hence, the ratio of their perimeters is $1 : \sqrt{2}$ .

Chung Kevin - 3 months, 4 weeks ago

Comparable Areas

1 solution

0 pending reports