This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
9 solutions
Moderator note:
Simple standard approach.
i − 9 9 9 9 = i 9 9 9 9 1 = i 2 ⋅ 4 9 9 9 + 1 1 = ( i 2 ) 4 9 9 9 ⋅ i 1 = ( − 1 ) 4 9 9 9 ⋅ i 1 = ( − 1 ) ⋅ i 1 = − i 1 = i i 2 = i
Log in to reply
Correct! I used an identity instead of rationalizing the expression.
i − 9 9 9 9 = i − 9 9 9 9 ∗ 1 2 5 0 0 = i − 9 9 9 9 ∗ ( i 4 ) 2 5 0 0 = i − 9 9 9 9 + 1 0 0 0 0 = i
i − 9 9 9 9 = i 9 9 9 9 1 = i 1 0 0 0 0 i = i
i^4 = 1, so i ^10000 = 1. i^(-9999) = i^(-9999)*i^10000 = i^1 = i.
i − 9 9 9 9 = i − 9 9 9 9 + 1 0 0 0 0 = i . Adding or subtracting any multiple of four does not change the value. So, add or subtract to/from the exponent, such a number that the result is 0, 1, 2, or 3 and we get the answer. OR F o r i n f i n d n ≡ x ( m o d 4 ) , i n = i x if you know mods.
Consider − 9 9 9 9 ≡ − 3 ( m o d 4 ) Which is no other than 1
Note that the powers of i cycle ever 4 powers. This means that the value of i depends on the module 4 of the power, where module 0 is just 1 . So, we have that i − 9 9 9 9 = i − 1 0 0 0 0 ⋅ i = 1 ⋅ i = i
- Let i 1 =x
- Then, 1=x i
- i 4 =x i
- x= i 3
- i 1 = i 3
- Therefore, ( i − 9 9 9 9 ) 1 = i
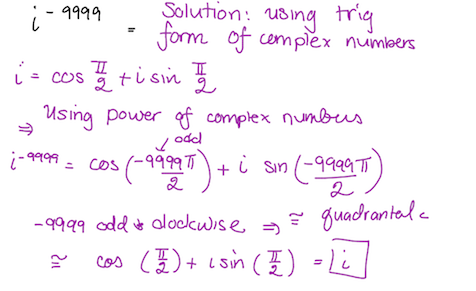
We have i − 9 9 9 9 = i 9 9 9 9 1 = i 4 ⋅ 2 4 9 9 + 3 1 = i 4 ⋅ 2 4 9 9 ⋅ i 3 1 .
Since i 4 = 1 , the equation can be simplified as
i 3 1 = − i 1 = − ( i 1 ) = − ( − i ) = i . □