Concurrent Cevians
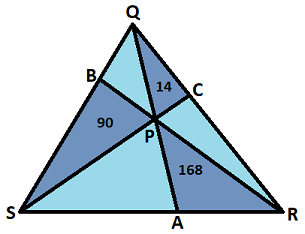
In , is the point of concurrency for cevians , , and so that the areas of , , and are , , and , respectively.
Find the area of .
The answer is 595.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
If the areas of A P S , B P Q and C P R are x , y , z respectively, the Ceva's Theorem tells us that 1 6 8 x × 1 4 z × 9 0 y = 1 so that x y z = 2 1 1 6 8 0 . Comparing the areas of A P S , A Q S , A P R and A Q R tells us that 1 6 8 x = z + 1 8 2 x + y + 9 0 and, similarly 1 4 z = y + 1 0 4 x + z + 1 6 8 9 0 y = x + 2 5 8 y + z + 1 4 Solving these various identities gives only one positive solution for x , y , z , namely x = 2 5 2 , y = 1 5 , z = 5 6 . This makes the area of the triangle Q R S equal to 5 9 5 .