Cone, Sphere, and Cylinder experiment

The volume of the cylinder is $V_{cylinder}=\pi r^2h$ , however, $h=2r$ . So it becomes $V_{cylinder}=2 \pi r^3$ .

The volume of the cone is $V_{cone}=\dfrac{1}{3} \pi r^2 h$ , however, $h=2r$ . So it becomes $V_{cone}=\dfrac{2}{3} \pi r^3$ .

After pouring some liquid from the cylinder to the cone (until the cone is full), the remaining volume of liquid is $2 \pi r^3-\dfrac{2}{3} \pi r^3=\dfrac{4}{3} \pi r^3$ , which is exactly the volume of the sphere.

$\large \boxed{\color{#D61F06}\mbox{Conclusion: There was exactly enough liquid left to fill the sphere}}$

The volume of water we have is the volume of the cylinder of a radius $R$ and height $2R$ , namely:

$V_{cyliner}= \pi R^2 \cdot 2R = 2\pi R^3$

The volume which is left after we pour the water into cone of radius $R$ and height $2R$ is:

$V_{cylinder} -V_{cone} = 2 \pi R^3 -\frac{1}{3} \pi R^2\cdot 2R= 2\pi R^3 -\frac{2}{3} \pi R^3 = \frac{4}{3} \pi R^3$

....which of course is the total volume of a sphere! We have no water left.

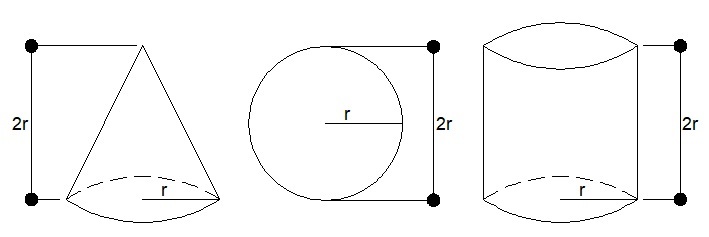One way to do this is to compare the volumes of the cylinder against the sum of the volumes of the sphere and cone. The volume of the cylinder is $\pi r^2(2r)=2\pi r^3$ . The sum of the volumes of the sphere and the cone is $\dfrac{4}{3} \pi r^3 + \dfrac{1}{3}\pi r^2(2r)=\dfrac{4}{3}\pi r^3 + \dfrac{2}{3}\pi r^3 = 2\pi r^3$ . Since the volumes are equal the desired answer is $\boxed{\text{There was exactly enough liquid left to fill the sphere }}$