Cone Surface Dynamics - Another Bird's Nest

See the first part (with solution) here .

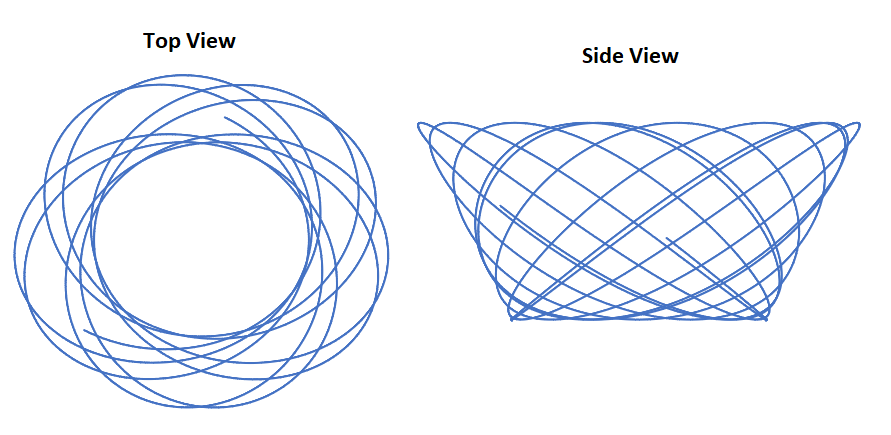
A massive particle is confined to the surface of a cone in the $xyz$ -coordinate system. The particle moves without energy losses over the surface of the cone: $\begin{aligned} x &= z \,\cos \theta \\ y &= z \, \sin \theta \\ z &= z \end{aligned}$ At time $t = 0$ (seconds), the particle's position and velocity are described as follows (SI units): $\begin{aligned} \theta &= 3.84847024468 \\ z &= 3.23159004274 \\ \dot{\theta} &= 1.52674026906 \\ \dot{z} &= -1.77089848408 \end{aligned}$ There is an ambient gravitational acceleration of $10 \text{ m/s}^2$ in the $-z$ (downward) direction. The system dynamics cause the particle to trace out a "bird's nest" pattern on the surface of the cone (similar to that of the previous problem, except now the envelope is clearly conical).

What is the particle's $x$ -coordinate (in meters) at time $t = 30$ (seconds)?

Note: This exercise requires numerical integration.