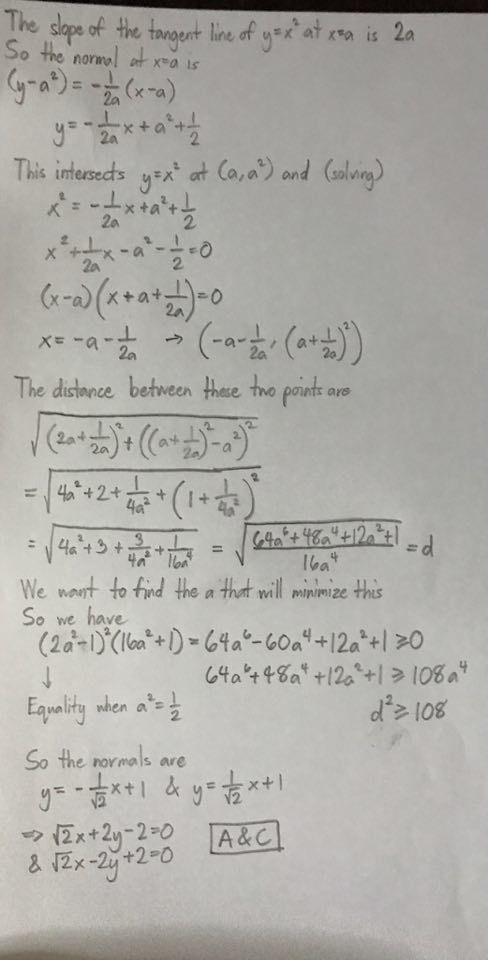Conics

Geometry Level 4

What normal to the curve y=x^2 forms the shortest chord?

  • A: √2x-2y+2=0
  • B: √2y+2x-2=0
  • C: √2x+2y-2=0
  • D: √2x+2y+2=0

Enter your answer as a 4 digit string of 1s and 9s, using 1 for correct option, 9 for wrong. For example, 1199 indicates A and B are correct, C and D are incorrect. None, one or all may also be correct.


The answer is 1919.

Vinay Jain by Vinay Jain , 22, India

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

2 solutions

2 Helpful 0 Interesting 0 Brilliant 0 Confused

A,B and C intersects X=0 at (0,1). However the slope of B is much steeper than that of A and C.
So B intersects Y= X 2 X^2 at much higher point. While B enters also at a point lower than those of A and C. A and B has the same value of the slope, they are symmetrically inclined to the axes of symmetry of Y= X 2 X^2 .
D cuts X=0 , axes of symmetry of Y= X 2 X^2 out side || C, never intersects the curve.
So A and C are Shortest chord.


0 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...