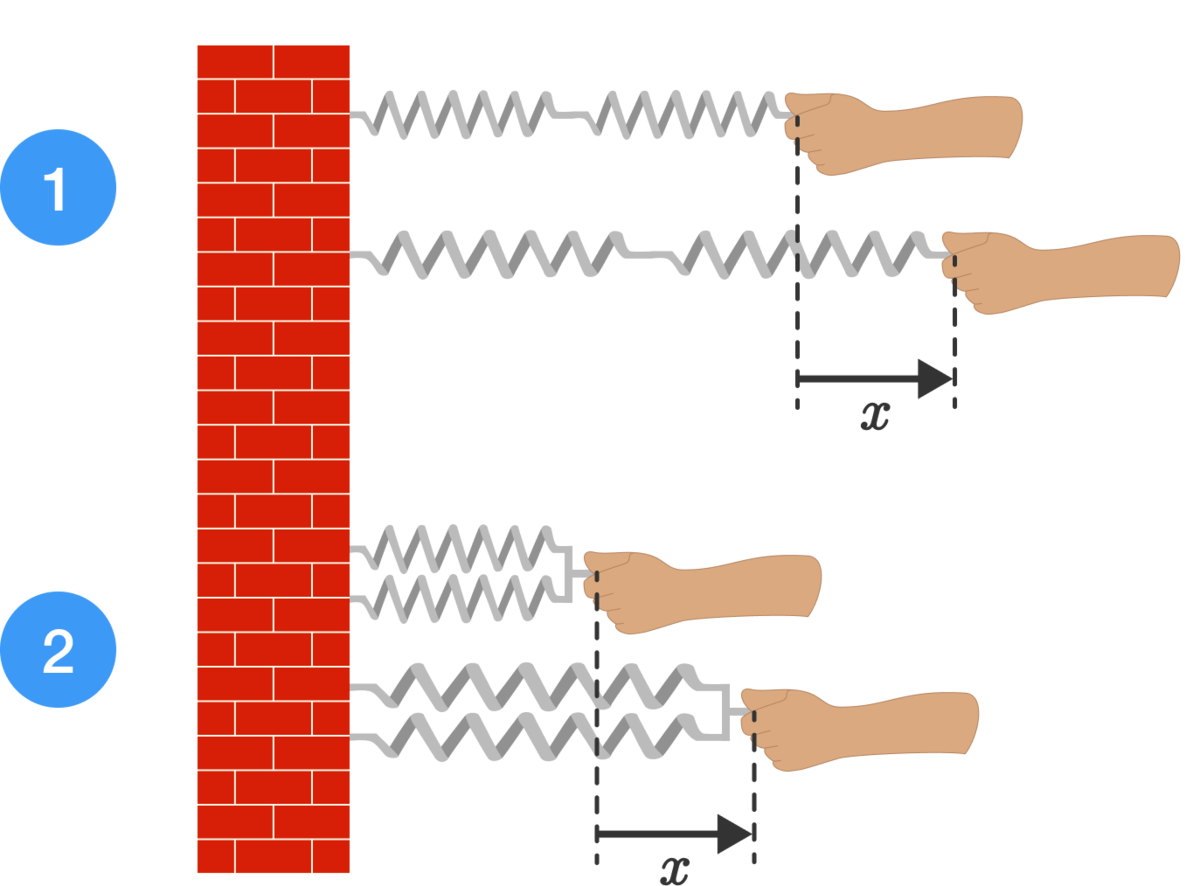
Connecting Springs

Let’s denote by $W$ the amount of work required to stretch a single spring by $x$ . Then in the second case, where the two springs are connected in parallel, each of them is displaced by $x$ . So the amount of work is $W_2 = 2W$ .

In the first case, where the springs are connected in series, each one of them needs only be stretched by $\frac x2$ , which requires only a quarter of the work $W$ . (This is because the potential energy of a single spring is quadratic in its displacement: $E(x)=\frac 12 kx^2$ , where $k$ is the spring's stiffness.) As we have two springs, we get $W_1 = 2\frac W4 = \frac W2$ . Therefore $\frac{W_1}{W_2}=\frac 14$ .