Consecutive is unpredictable (Part 2)
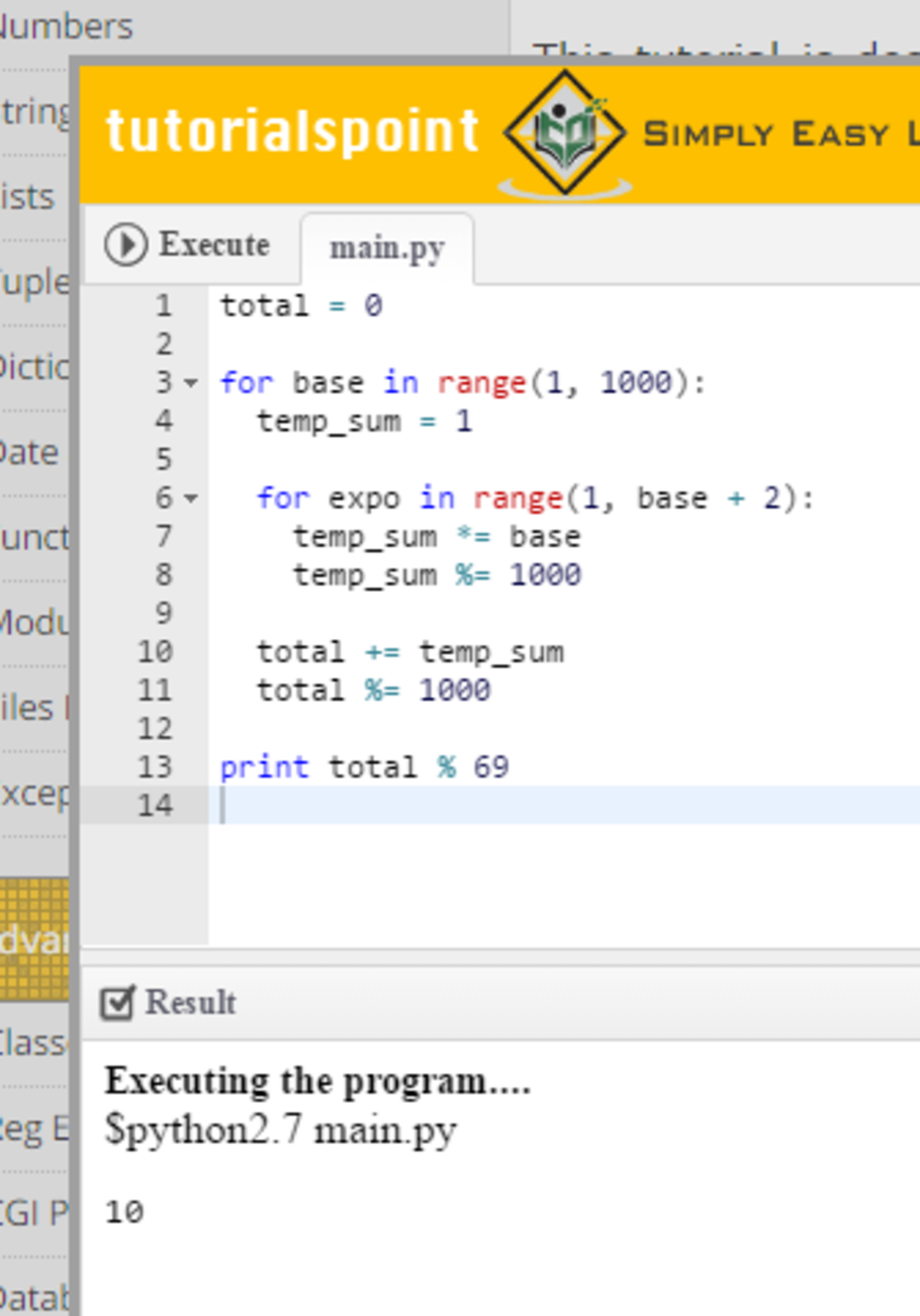
Let be the last three digits of .
Find .
Details:
The exponents are in a consecutive order of the positive integers:
is three different digits, like , not algebraic expression like
More challenging problems? Here!
The answer is 10.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
effortless :) (Frequent use of modulo prevents the variable to balloon in value)