Constructing a Platonic solid
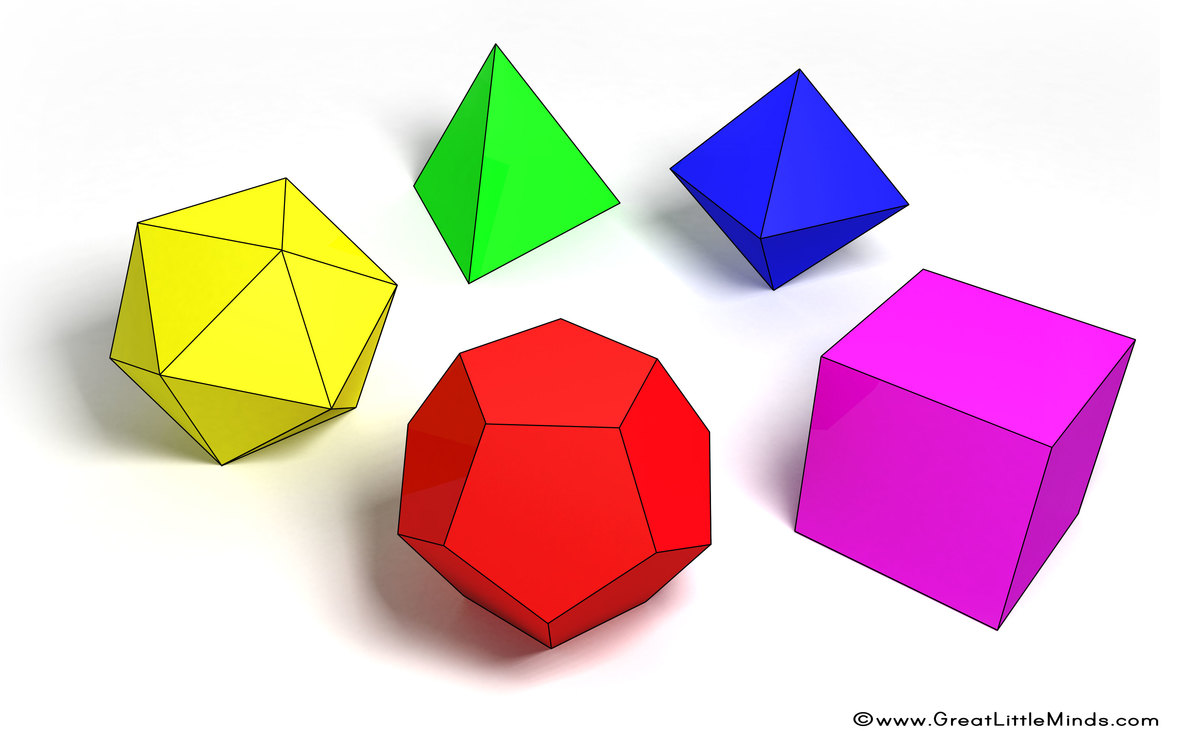
You have a collection of Platonic solids each having an edge length of one, Lets call these "unit Platonic solids". You realize that you can glue them all together to construct a much larger Platonic solid leaving no gaps or holes.
In your final construction (one large Platonic solid) you have used exactly 364 of one type of the unit Platonic solids, but may have used other types of unit Platonic solids as well.
How many unit Platonic solids did you use altogether in this final construction?
For example, if your final consturction is a Platonic solid mad up of 364 unit cubes and 212 unit tetrahedra, then your final answer would be 576.
Inspiration: Michael Mendrin
Image credit: www.greatlittleminds.com
The answer is 1105.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
There are five Platonic solids: Octahedron, Cube, Tetrahedron, Icosahedron, and Dodecahedron.
The only two Platonic solids that can be formed from different Platonic solids are the tetrahedron and the octahedron. (The cube is out, since 364 isn't a cubic number)
The formulae for constructing these solids (thanks @Michael Mendrin for providing these!) are as follows:
To make tetrahedra of linear size n , you need
For example for n = 2 we have this construction:
To make octahedra of linear size n , you need
For example, for n = 2 , we have this construction:
For integer n , the only equation which gives 3 6 4 of any of these is the first one.
This is for n = 1 3 , which implies you have 3 6 4 ocathedra and 7 4 1 tetrahedra and you are constructing a large tetrahedron.
So the total number of Platonic solids you begin with is 3 6 4 + 7 4 1 = 1 1 0 5