Construction Is A Piece Of Cake! Right? (Part-4)

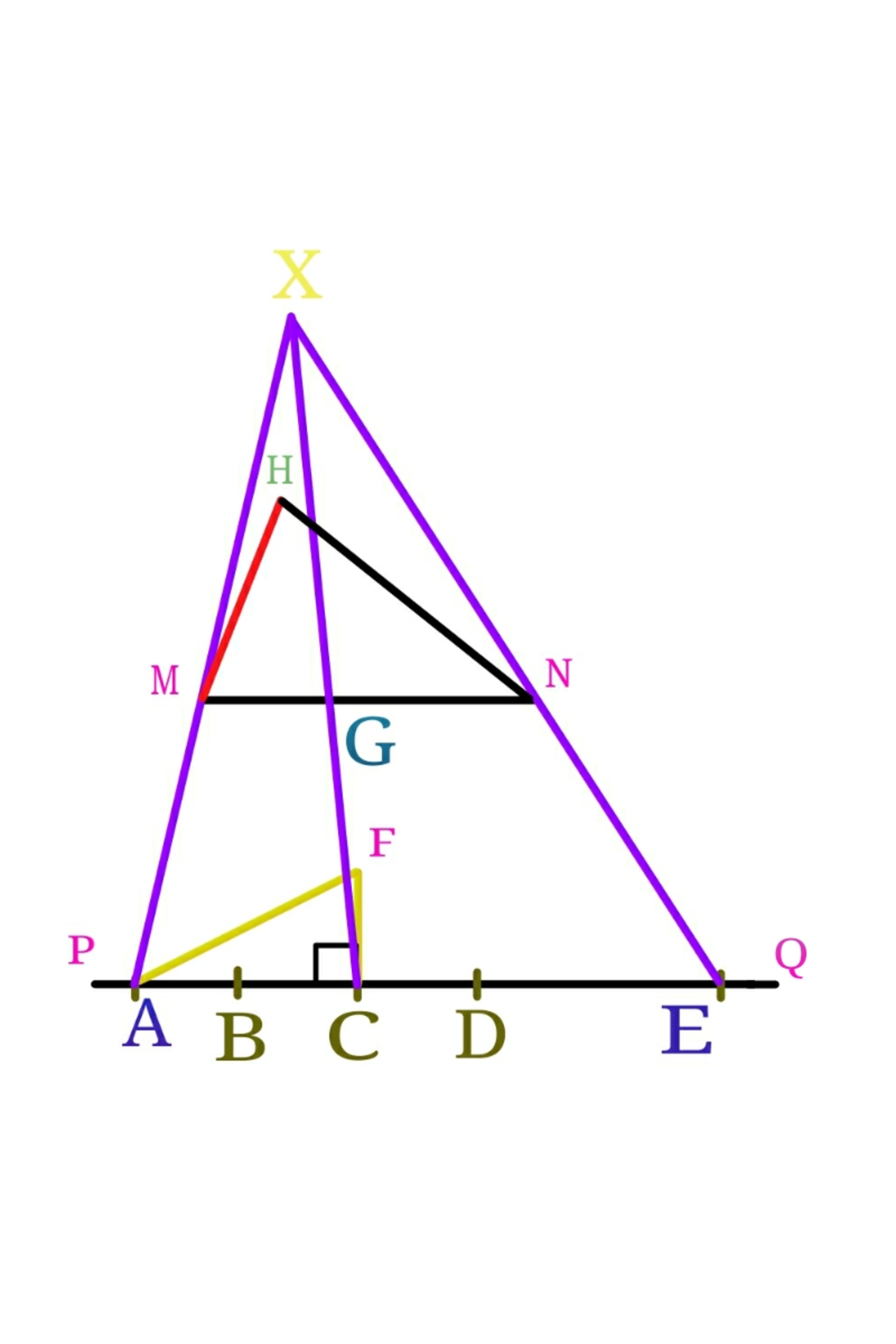
Sanskar, influenced by Mohit , Akshat and Anshuman , took two parallel lines $PQ$ and $MN$ (as shown in the figure) such that $MN<PQ$ , and started doing aimless constructions, the steps of which are given below:

( $1$ ) He marked four points $A, B, C \mathrm{ and } D$ such that $AB=BC=CD$ and then he drew a perpendicular on $C$ and marked a point $F$ on it such that $AB=CF$ .

( $2$ ) Then he joined $AF$ and marked a point $E$ on $PQ$ such that $DE=AF$ and $E$ doesn't lies on $PD$ .

( $3$ ) He then joined $EN$ which meets $AM$ at $X$ (both lines extended).

( $4$ ) Then he joined $CX$ which intersects $MN$ at $G$ and then constructed $\bigtriangleup MHN$ such that $MH=GN$ and $HN=MN$

Sanskar then wondered what $\angle MNH$ could possibly be equal to (in degrees).