Count 'em All 20!
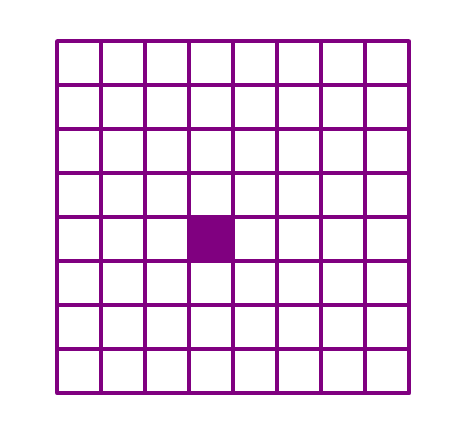
The figure above shows an grid but with a hole in it.
Count the total number of quadrilaterals in the grid above.
Clarifications :
- A quadrilateral is a polygon that has 4 sides.
- The quadrilaterals cannot contain the hole! I don't like holes.
This is one part of Quadrilatorics .
The answer is 896.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
All of the rectangles(including those containing the hole): ( 1 + 2 + . . . + 8 ) 2 = 1 2 9 6
The ones containing the hole:The rectangle is formed with 2 horizontal lines and 2 vertical lines.
One of the horizontal lines must be on the left of the hole(4 ways),the other is on the right of the hole(5 ways).
One of the vertical lines must be above the hole(5 ways),the other is below the hole(4 ways).
So there are 4 × 5 × 5 × 4 = 4 0 0 rectangles containing the hole.
Hence,there are 1 2 9 6 − 4 0 0 = 8 9 6 rectangles not containing the hole.