Cow pasture

This problem is special case for an isoperimetric problem. The shape, that maximizes the area for a given perimeter, is a circle. Therefore, we can sketch the solution as follows: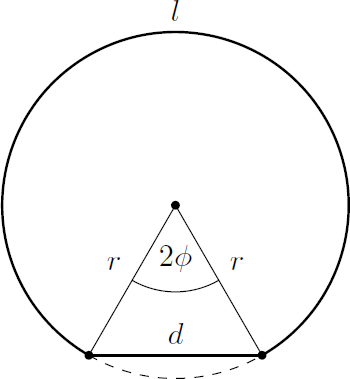 For a circle with radius $r$ and a triangular segement with angle $2 \phi$ we can rewrite the quantities $d$ and $l$ by $\begin{aligned} d &= 2 r \sin \phi \\ l &= 2 (\pi - \phi) r \\ \Rightarrow \quad \frac{d}{l} &= \frac{\sin \phi}{\pi - \phi} \approx 0.19099 \end{aligned}$ The numerical solution for $\phi$ results $\phi \approx 0.523609 \approx 30^\circ$ Therefore, $r \approx d$ and $A = (\pi - \phi + \sin \phi \cos \phi) r^2 \approx \left( \frac{5}{6} \pi + \frac{\sqrt{3}}{4} \right) r^2 \approx 5589\,\text{m}^2$