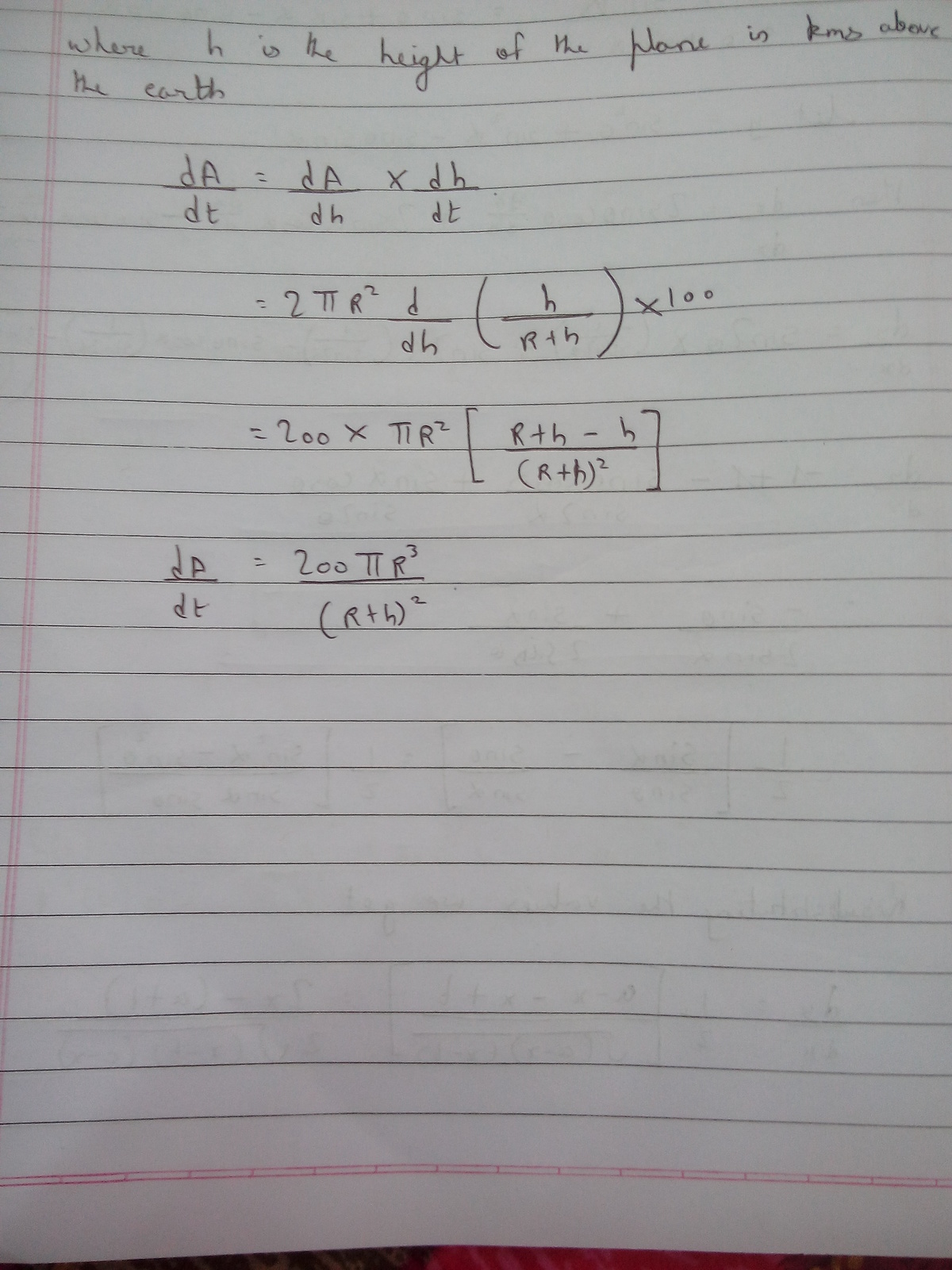Crazy Calculus 6
An air force plane is ascending vertically at the rate of 1 0 0 km/hr . If the radius of the Earth is R km , how fast is the area of the Earth visible from the plane increasing at 3 minutes after it started ascending?
Take visible area A = R + H 2 π R 2 H , where H is the height of the plane (in km ) above the Earth.
P : ( R + 5 ) 2 2 0 0 π r 3 h k m 2
Q : ( R + 5 ) 2 0 0 π r 3 h k m 2
R : ( R + 5 ) 4 0 0 π r 3 h k m 2
S : ( R + 5 ) 2 4 0 0 π r 3 h k m 2
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.