This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
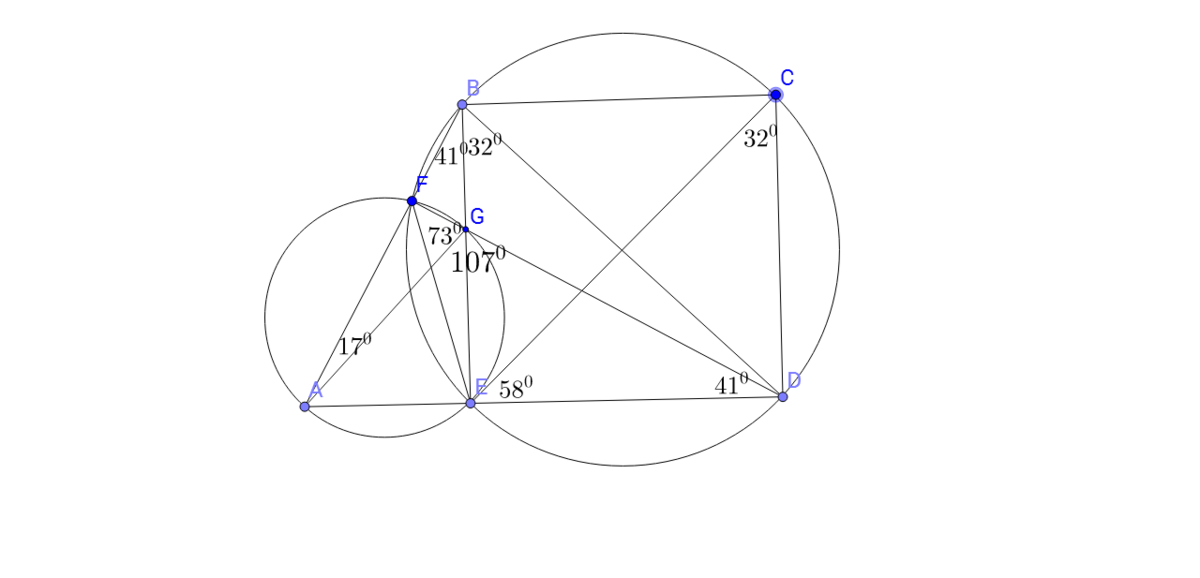
Observe that B F E D , A F G E , B C D E are all cyclic quadrilaterals.
In B F E D , we have ∠ F D E = ∠ F B E = 4 1 0 .
Also, in B C D E , we have ∠ D C E = 3 2 0 = ∠ D B E .
Now observe that in △ A B D D F and B E are altitudes intersecting at G .
Hence, G is the orthocenter of △ A B D which implies ∠ A G D = 1 8 0 0 − ∠ A B D = 1 8 0 0 − ( 4 1 0 + 3 2 0 ) = 1 0 7 0 .
As F G D is a straight line, we have ∠ A G D + ∠ A G F = 1 8 0 0 ⇒ ∠ F G A = 7 3 0 .
Applying angle sum rule in triangle F A G , we get ∠ G A F = 1 7 0 .
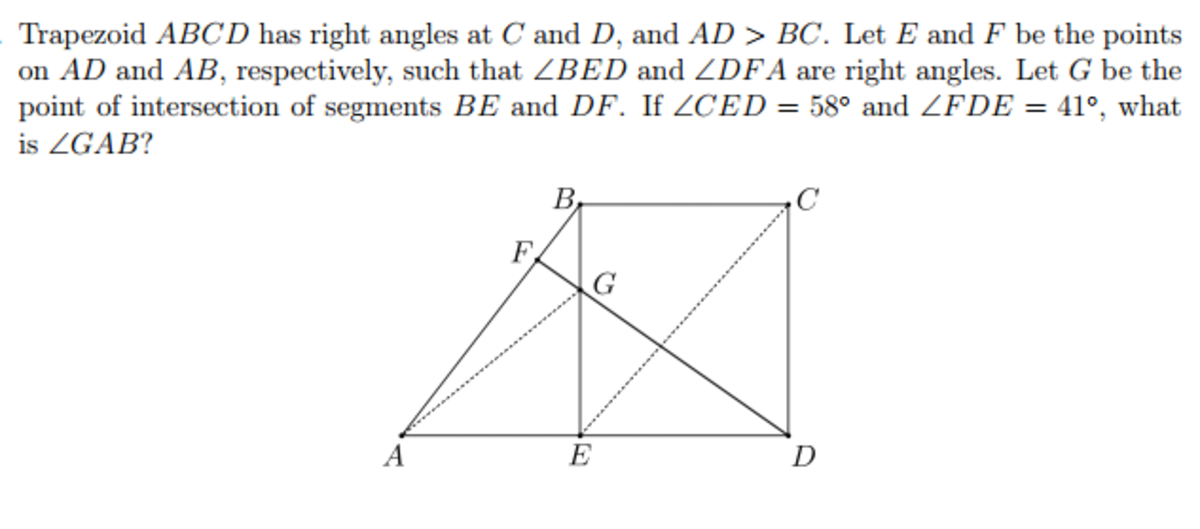
Join FE, and FC . Since BCDE, is a rectangle, so BCDE is a circle. In quadrilateral BCDF sum of opposite angles ∠ D F B + ∠ B C D = 1 8 0 . ∴ i t i s c y c l i c . ⟹ B C D E F i s a c i r c l e . ∠ C E B = ∠ D E B − ∠ D E C = 3 2 . A n g l e s o n c h o r d F C , ∠ C D F = ∠ C E F , ⟹ ∠ C D E − ∠ F D E = ∠ C E B + ∠ B E F ⟹ 9 0 − 4 1 = 3 2 + ∠ B E F . ∴ ∠ B E F = 1 7 . I n q u a d r i l a t e r a l A F G E , ∠ s a t F , a n d E a r e 9 0 o , ∴ i t i s c y c l i c . ∴ ∠ G A B = ∠ B E F = 1 7