Cube / Octahedron Surface Area
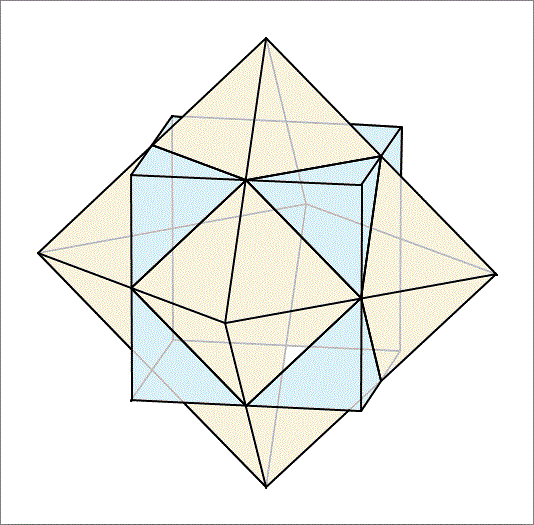
A cube and an octahedron are superimposed to create the polyhedron depicted in the figure above.
If the side length of the cube is , what the surface area of this polyhedron ? The answer can expressed as for positive integers , with square-free. Find .
The answer is 9.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Each of the blue triangles is an isosceles right triangle with legs of 2 1 , a hypotenuse of 2 2 , and an area of B = 2 1 ⋅ 2 1 ⋅ 2 1 = 8 1 .
Each of the yellow triangles is an equilateral triangle with sides of 2 2 and an area of Y = 4 3 ⋅ ( 2 2 ) 2 = 8 3 .
There are 2 4 blue and 2 4 yellow triangles, so the surface area is S = 2 4 B + 2 4 Y = 2 4 ⋅ 8 1 + 2 4 ⋅ 8 3 = 3 + 3 3 .
Therefore, a = b = c = 3 and a + b + c = 9 .