Cubing the Sphere

Geometry Level 3

A cube and a sphere have the same volume, and have the same center (see figure). If the side length of the cube is 40 cm, find the volume (in cubic centimeters) of one of the spherical caps (the portions of the sphere that lie outside the cube).

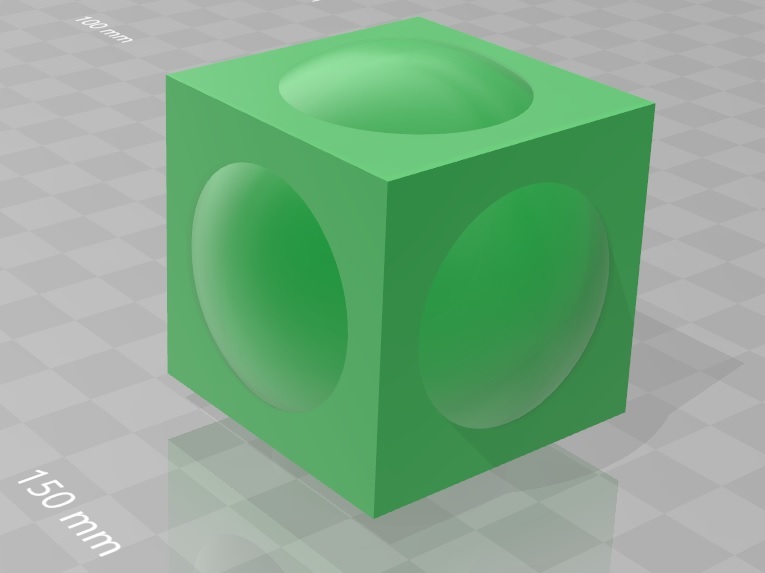
(Image Created with Microsoft 3D Builder)


The answer is 1689.8.

Hosam Hajjir by Hosam Hajjir , 56, Canada

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

1 solution

Parth Sankhe
Oct 10, 2018

4 3 π r 3 = 4 0 3 \frac {4}{3}πr^{3} = 40^{3}

Volume of a spherical cap is given by

π h 2 ( 3 r − h ) 3 \frac {πh^{2}(3r-h)}{3}

Here h = r − 40 2 h = r - \frac {40}{2} (height of the spherical cap)

By putting the values we get V = 1689.09 V=1689.09

3 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...