Curvilinear Asymptote

This shows the correct curvilinear asymptote ${y}^{3}=x$ superimposed over the implicit function plot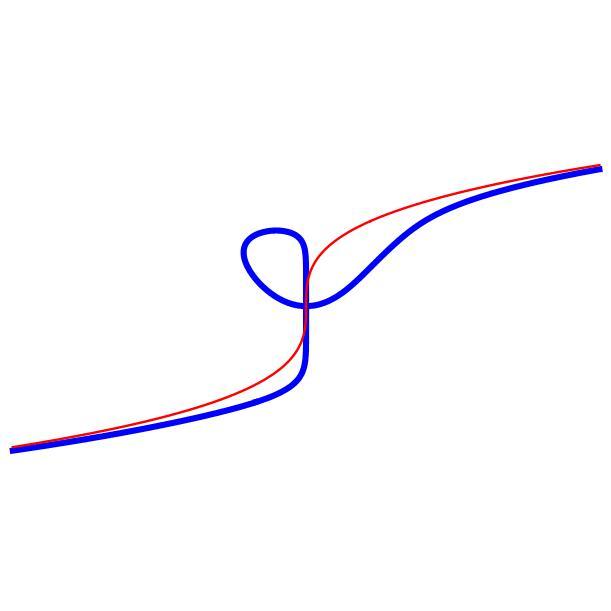

The first step is to find the "improper asymptote", by first rearranging the implicit equation to as follows

$\left( { y }^{ 3 }-x \right) \left( { y }^{ 6 }+{ x }^{ 2 } \right) +xy=0$

then scaling this function in this fashion $y \rightarrow ky$ and $x \rightarrow {k}^{3}x$ gets us

$\left( { y }^{ 3 }-x \right) \left( { y }^{ 6 }+{ x }^{ 2 } \right) +\dfrac { xy }{ { k }^{ 9 } } =0$

which means that the last term drops out as $k \rightarrow \infty$ and the first term which contains ${y}^{3}-x=0$ becomes dominant. This this at least the improper asymptote, i.e, this is what the implicit curve looks like in the far field. The next step is proving that the distance between this improper asymptote and the implicit curve approaches $0$ as the limit, which is a necessary step to showing that this asymptote is a proper one.

To verify that this is a proper asymptote, from a cursory examination of the proposed asymptote, we assume that $y={x}^{\frac{1}{3}}-a$ , where $a$ is the vertical distance from asymptote to the implicit curve. Then we have

$\left( {({x}^{\frac{1}{3}}-a) }^{ 3 }-x \right) \left( {({x}^{\frac{1}{3}}-a) }^{ 6 }+{ x }^{ 2 } \right) +x({x}^{\frac{1}{3}}-a)=0$

which, after expanding and rearranging, we have

$6a{x}^{\frac{4}{3}}=1+a({x}^{p_i})$

where $p_i \le 1$ . Thus, for large $x$ , this last term drops off, and we're left with

$6a{x}^{\frac{4}{3}}=1$

so that as $x \rightarrow \infty$ , $a \rightarrow 0$ , and we know now that ${ y }^{ 3 }-x =0$ is a proper asymptote.