Cutting A Hexagon
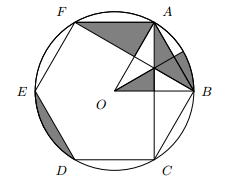
The figure above shows a regular hexagon inscribed inside a circle with center such that . Find the area of the shaded region.
If the area can be written as , where and are integers with both primes , submit your answer as .
The answer is 13.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
A C and F B are perpendicular bisectors of △ A B O ⇒ the areas b and e are equals. Then b + c + d is equal to 2 1 of △ A B O area. Also b + c + d = a because O A is perpendicular bisector of O A ∴ a + b + c + d is equal to △ A B O area.
△ A B O area is equal to 4 3
Area g is two times area f . Area g is equal to a sixth part of the circle's area minus △ A B O area. This is 6 π − 4 3 = 1 2 2 π − 3 3 ⇒ g + f = 1 . 5 ( 1 2 2 π − 3 3 ) = 8 2 π − 3 3
a + b + c + d + e + f + g = 8 2 π − 3 3 + 4 3 = 8 2 π − 3
Be free of asking any question in comments