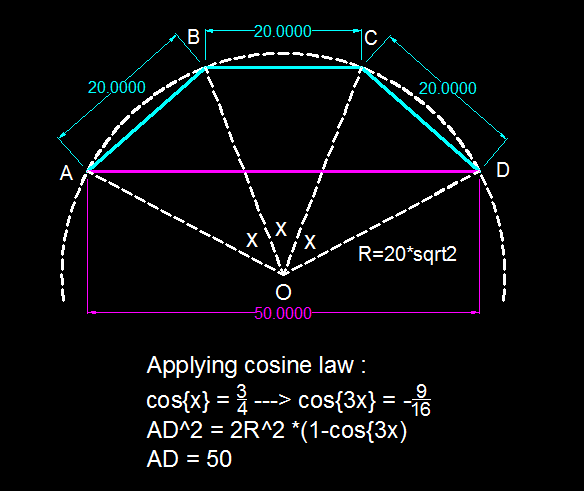
Cyclic Quadrilateral

Non-trig solution:

Note: There are at least seven ways to solve this. This is just the way I solved it.

Let $O$ be the center of the circle, and $x$ be the shorter altitude of $\triangle OBC$ . The length of $x$ is $\dfrac{1}{2}$ the length of $AC$ .

By the Pythagorean Theorem, the longer altitude of $\triangle OBC$ is $10\sqrt{7}$ . We can therefore compute the area of $\triangle OBC$ to be $100\sqrt{7}$ .

Using the formula for the area of a triangle,

$\dfrac{1}{2}\cdot20\sqrt{2}\cdot x =100\sqrt{7}$

Solving for $x$ yields $x=5\sqrt{14}$ . Therefore, the length of $AC$ is $10\sqrt{14}$ .

By Ptolemy's Theorem ,

$10\sqrt{14}\cdot10\sqrt{14}=20\cdot20+20\cdot AD$

$1400=400+20\cdot AD$

$AD=\boxed{50}$