#Cyclic quadrilateral
In a cyclic quadrilateral A B C D , A B = 3 , B C = 4 , C D = 5 , D A = 6 , and A E is a diameter of its circumcircle. If sin ∠ C A E = n m , where m and n are positive coprime integers, enter m + n .
The answer is 10.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
Draw the center O and △ A O C :
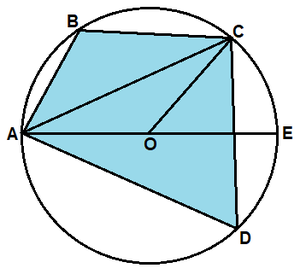
By the properties of a cyclic quadrilateral , cos ∠ D = 2 ( a d + b c ) a 2 + d 2 − b 2 − c 2 = 2 ( 5 ⋅ 6 + 3 ⋅ 4 ) 5 2 + 6 2 − 3 2 − 4 2 = 7 3 .
As a central angle intercepting the same arc of inscribed angle ∠ D , ∠ A O C = 2 ∠ D .
As a base angle of isosceles triangle △ A O C , ∠ C A E = 2 1 ( 1 8 0 ° − ∠ A O C ) = 9 0 ° − ∠ D .
Therefore, sin ∠ C A E = sin ( 9 0 ° − ∠ D ) = cos ∠ D = 7 3 , so m = 3 , n = 7 , and m + n = 1 0 .
Let the side lengths of quadrilateral A B C D be a = 3 , b = 4 , c = 5 , and d = 6 . By Ptolemy's theorem , the diagonal A C :
q = a b + c d ( a c + b d ) ( a d + b c ) = 7 2 4 7
The diameter A E of a circumcircle is given by
D = sin B A C = sin B q ⟹ sin B = D q
Since A E is a diameter, by Thales theorem we have ∠ A C E = 9 0 ∘ . Let ∠ C A E = θ . Then
sin θ = A E C E = A E A E 2 − A C 2 = D D 2 − q 2 = 1 − ( D q ) 2 = 1 − sin 2 B = − cos B Since ∠ B is obtuse,
By cosine rule ,
q 2 ⟹ cos B ⟹ sin θ = a 2 + b 2 − 2 a b cos B = 2 a b a 2 + b 2 − q 2 = 2 ⋅ 3 ⋅ 4 ⋅ 7 7 ( 3 2 + 4 2 ) − 2 4 7 = − 7 3 = − cos B = 7 3
Therefore m + n = 3 + 7 = 1 0 .