Dancing Shapes

Probability Level 4

A dance club has set up a social mixer on a “Lucky for Some!” singles night that is designed to overcome shyness and allow people to find someone interesting. On the dance floor they have marked a giant triangle that has been divided into 64 internal triangular cells as shown in the figure. At the start of the dance, 64 ticket holders are each randomly assigned a cell. Then the emcee plays a popular song whose lyrics are 13 lines long. While a line is being sung, participants have to dance in the cell that they find themselves in. At the end of each line, a drumbeat signals that each dancer must move across one of the lines bordering the cell into an adjacent cell. At the end of the song, the lucky dancers whose originally assigned cells are now empty can choose any partner, and the couple is given drinks on the house. (Thirteen, it appears, can be lucky for some!)

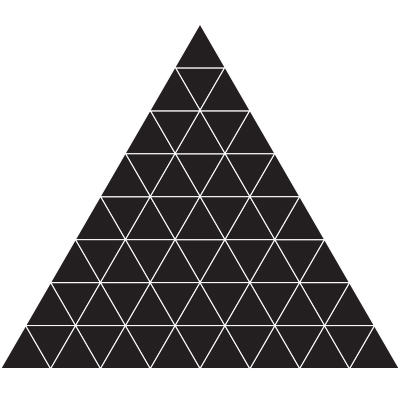What is the minimum number of winners -- the dancers for whom the club will have to buy drinks -- if the dancers follow the instructions correctly?

Resource: Quanta Magazine

The answer is 8.

1 solution

Brian Lie
May 28, 2019

We color all the upward pointing triangles light and those pointing downward dark. As you can see, there are no neighboring cells of the same color. At the end of each of the song’s 13 lines, every dancer has to switch parity, going from a dark-colored cell to a light-colored cell and vice-versa. After an odd number of rounds, all the dancers that started on light cells will have to be on dark cells, no matter what adjacent cells they chose each time they switched. The number 13 is a red herring here -- it could have been any odd number. There are 36 light cells and 28 dark cells, so after every odd round, and therefore at the end of the song, at least 8 light cells have to be empty.

On the other hand, 8 can be achieved as Mark Hennings' comment below. Since each of the dancers whose original cells were empty could possibly choose another from this group to have drinks with, the minimum number of winners is $\boxed 8$ .

Try to problem Dancing Shapes II .

Brian Lie - 2 years ago

Nice solution! (And nice problem too)

Chris Lewis - 2 years ago

Glad you enjoyed it.

Brian Lie - 2 years ago

It is worth showing that $8$ can be achieved. Any two partners who share a "horizontal" edge just swap squares each time. This means that all triangles will always be full, except possibly for the bottom eight light triangles. The people who start in the bottom eight light triangles can do anything they like - after 13 swaps they will be in dark triangles, so the bottom eight light triangles will be empty.

Mark Hennings - 2 years ago

Thank you for your comment, sir.

Brian Lie - 2 years ago

Dancing Shapes

The answer is 8.

1 solution

0 pending reports