Dedicated to Ayush Rai
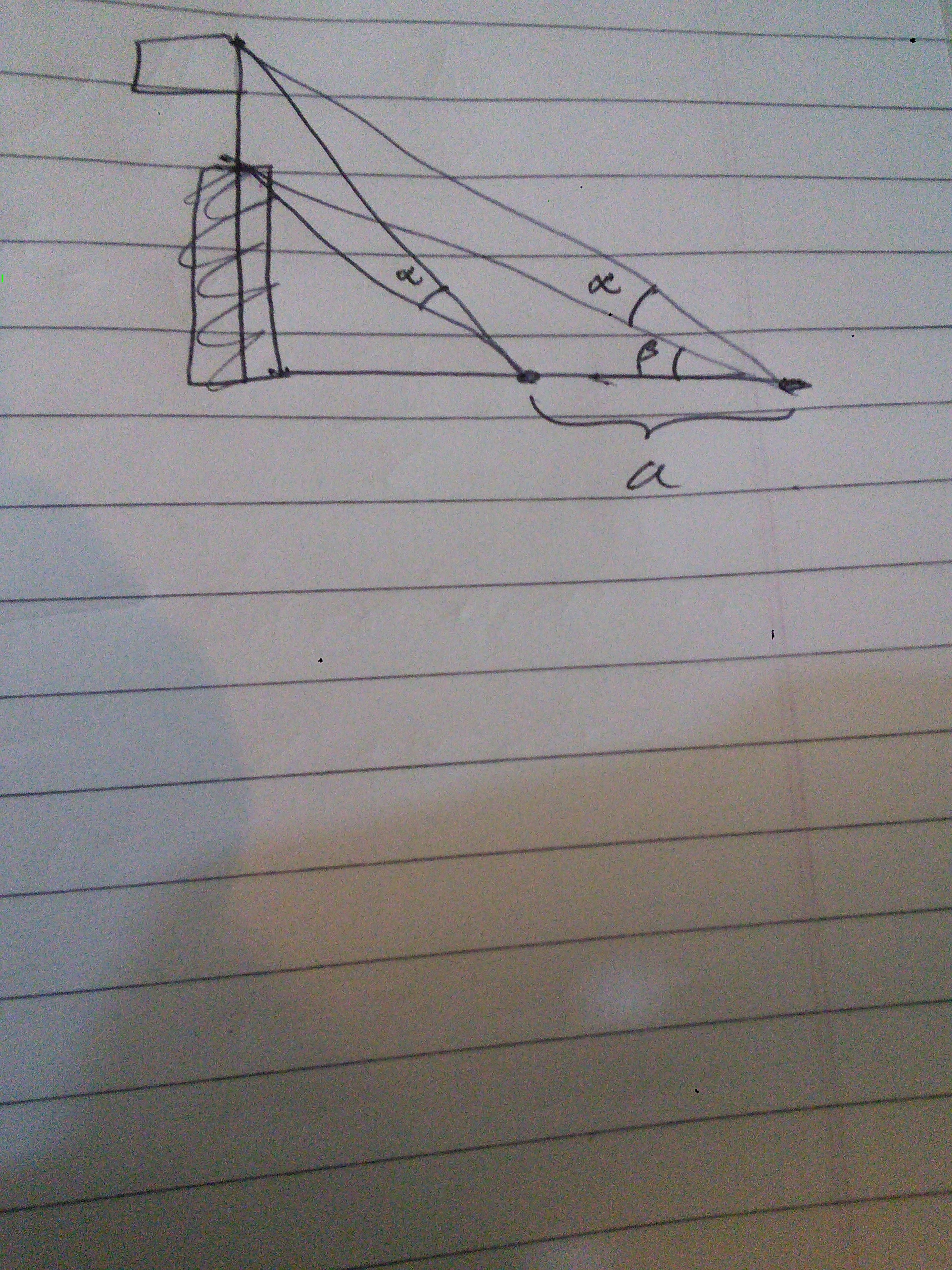
A flagstaff is on the top of a tower which stands on a horizontal plane.
A person observes the angles subtended by the flagstaff and the tower at a point on the horizontal plane as and respectively.
He then walks a distance towards the tower and observes that the angle subtended by the flagstaff remains unchanged.
Enter the height of the tower correct to three decimal places.
Details and assumptions:
Clarification figure:
Bonus questions:
- Generalise for arbitrary values of and .
- Find the height of the flagstaff in this generalised situation.
The answer is 2.732.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.

Let the point from where he first observes the tower and the flagstaff be A . Let the tower be represented by P Q and the flagstaff by P R . Also, let the second point of observation be B .
Then, α = ∠ P A R = ∠ P B R ( Given ) β = ∠ P A Q ( Given ) a = A B ( Given ) θ = ∠ B P A ( Say )
Note that the quadrilateral A B P R is concylic (this follows from the first equality).
Hence the following equalities hold: β = ∠ P A Q = ∠ B R Q θ = ∠ B P A = ∠ A R B
Now, by the exterior angle property, ∠ A P R = ∠ A Q P + ∠ P A Q = 9 0 ∘ + β
As the sum of angles in Δ A P R is 1 8 0 ∘ , θ = 9 0 ∘ − ( α + 2 β )
Applying the sine rule to appropriate triangles, we get sin ( θ + β ) A P = sin θ a = sin α P R
∴ Height of tower = P Q = A P sin β = a cos ( α + 2 β ) sin β cos ( α + β ) ∴ Height of flagstaff = P R = a cos ( α + 2 β ) sin α
Note: This problem and its solution have been taken from this book .