diagonals . . .
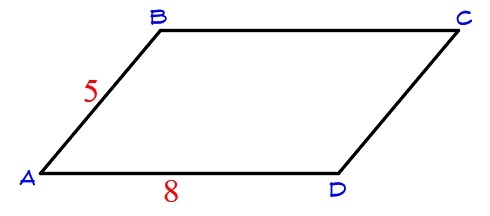 Shown above is a parallelogram. Given that
A
B
=
5
and
A
D
=
8
, find
(
B
D
)
2
+
(
A
C
)
2
.
Shown above is a parallelogram. Given that
A
B
=
5
and
A
D
=
8
, find
(
B
D
)
2
+
(
A
C
)
2
.
The answer is 178.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
The sum of the squares of the diagonals of a parallelogram equals the sum of the squares of its sides. In mathematical notation, ( A B ) 2 + ( B C ) 2 + ( C D ) 2 + ( A D ) 2 = ( B D ) 2 + ( A C ) 2 Therefore, ( B D ) 2 + ( A C ) 2 = 2 5 + 6 4 + 2 5 + 6 4 = 1 7 8
Join B D and C A
Applying cosine rule on triangle B A D
B D 2 = A B 2 + A D 2 + 2 . A B . A D . c o s A [ Equation 1 ]
Similarly, for triangle A D C
A C 2 = A D 2 + C D 2 + 2 . A D . C D . c o s D
Now note A B = C D = 5 A D = B C = 8 And angle D = 1 8 0 − A
So A C 2 = A D 2 + C D 2 + 2 . A D . 2 . A D . C D . c o s D becomes
A C 2 = A D 2 + A B 2 + 2 . A D . A B . c o s ( 1 8 0 − A ) [ Equation 2]
Note c o s ( 1 8 0 − A ) = − c o s A
Adding equation 1 and 2
B D 2 + A C 2 = 2 ( A B 2 + A D 2 ) + 2 A D . A B . c o s A − 2 . A D . A B . c o s A
B D 2 + A C 2 = 2 ( 5 2 + 8 2 )
= 1 7 8
NOTE: All the angles are in degrees