South, East, North?
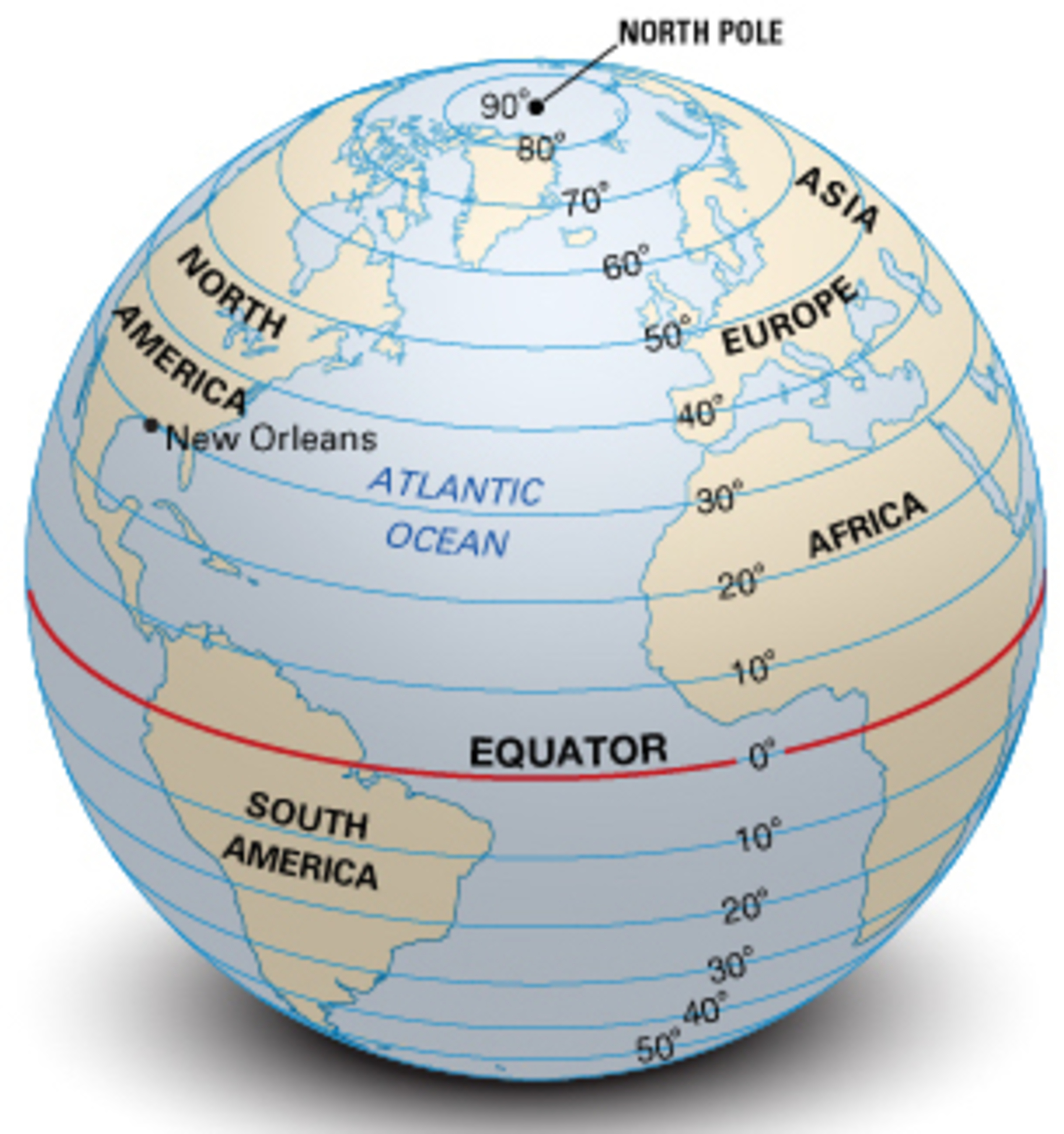
You go south 10 miles, east 10 miles, and then north 10 miles, and you end up in the same place you started. How many different degrees latitude could you have started from?
Clarification: The blue lines above represent different lines of latitude.
Image credit: Encyclopedia Britannica.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Well the obvious place is the north pole.
However, you could also go down toward the south pole, and if you start at a position where if you go 1 0 miles south, then going east for 1 0 miles is essentially walking in a circle and returning to where you started (i.e. the line of latitude at that point is a circle with a 10 mile circumference), then the above would also be satisfied. Its also satisfied if you pick a position such that once you walk 1 0 miles south, going west returns you to where you started after going 5 miles, so doing 2 such loops would be 1 0 miles. And similarly you can choose infinitely many positions chosen such that after going 1 0 miles south you "encircle the globe" n times when you walk 1 0 miles east, since this will work for any arbitrary value of n .
Note, the solution set consists of one point at the north pole, and then any number of points (a little more than 1 0 miles north of the south pole) chosen such that when you go south 1 0 miles you wind up at a spot where you will "encircle the earth" an integral number of times in 1 0 miles, so walking north again 1 0 miles puts you back where you started.