did i miss something XD (try this)

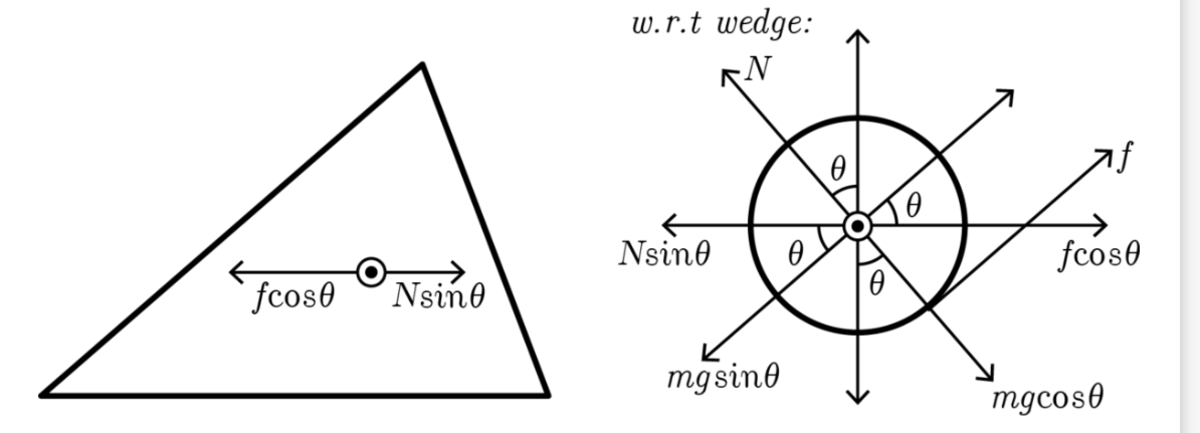
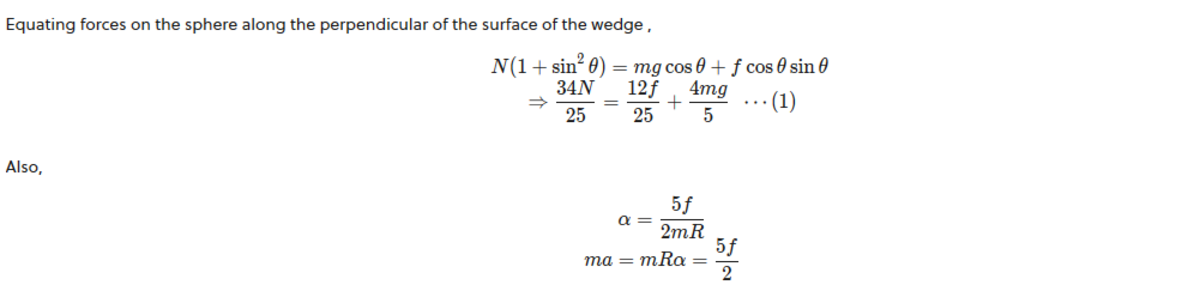
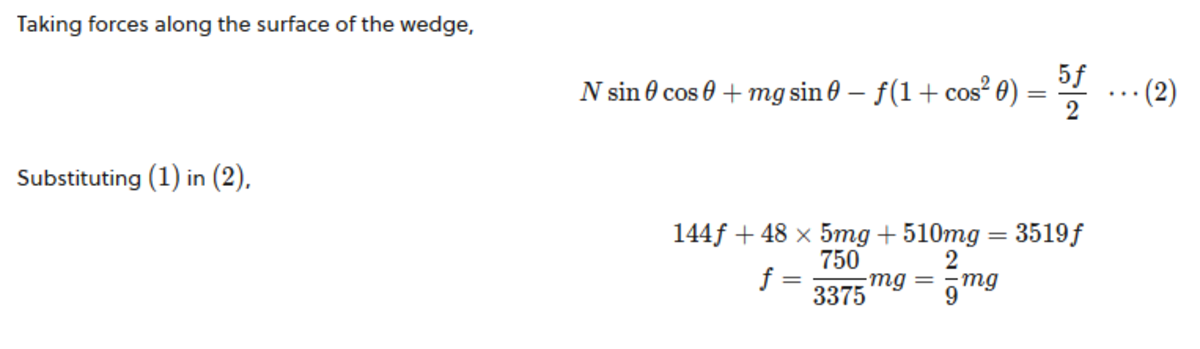
The force $ma_1$ acting on the sphere is a pseudo force.

The equations governing the motion of the sphere and wedge are:

$mg + N_2\cos{\theta} + f\sin{\theta} = N_1 \ \dots (1)$ $N_2\sin{\theta} - f\cos{\theta} = ma_1 \ \dots (2)$ $mg\cos{\theta} = N_2 + ma_1\sin{\theta} \ \dots (3)$ $ma_1\cos{\theta} + mg\sin{\theta} - f = ma_2 \ \dots (4)$ $fR = \left(\frac{2}{5}mR^2\right)\alpha \ \dots (5)$ $a_2 = R\alpha \ \dots (6)$

• Equation 1 balances forces along the vertical for the wedge.

• Equation 2 is the application of Newton's 2nd law for the wedge along the horizontal.

• Equation 3 is a force balance in a direction perpendicular to the incline for the sphere.

• Equation 4 is the application of Newton's 2nd law for the sphere along the incline.

• Equation 5 is the torque equation $\tau = I\alpha$ about the COM of the sphere.

• Equation 6 is the pure rolling constraint.

Taking $\cos{37^o}=0.8$ and solving the six equations in six unknowns gives:

$f = \frac{2}{9}mg$ $N_2 = \frac{2}{3}mg$

The resultant force between the wedge and the sphere is:

$F = \sqrt{f^2 + N_2^2}$

Apologies for the messy diagram. I have denoted $f$ as the friction force between the sphere and the wedge.