A geometry problem by Syed Hamza Khalid
Inside parallelogram A B C D , there are four yellow regions with areas 8, 10, 72, and 79, as shown.
Find the area of the red triangle.
Notes:
- The diagram is not drawn to scale
- The problem can be solved with basic knowledge of arithmetic and the formulas for the areas of parallelogram and triangle.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
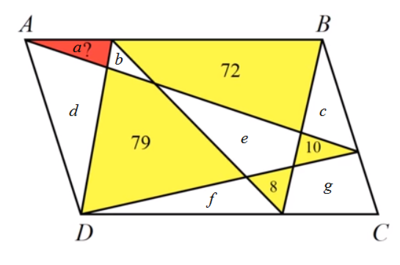 Assign the unknown areas as
a
,
b
,
c
to
g
as shown in the figure. Let the area of parallelogram
A
B
C
D
be
A
. We need to find
a
. We note that:
Assign the unknown areas as
a
,
b
,
c
to
g
as shown in the figure. Let the area of parallelogram
A
B
C
D
be
A
. We need to find
a
. We note that:
{ a + b + 7 2 + c + f + 8 + g = a + b + c + f + g + 8 0 = 2 1 A b + 7 9 + f + g + 1 0 + c = b + c + f + g + 8 9 = 2 1 A . . . ( 1 ) . . . ( 2 )
Note that ( 1 ) = ( 2 ) = 2 1 A :
⟹ a + b + c + f + g + 8 0 ⟹ a = b + c + f + g + 8 9 = 9
Hey Chew-Seong Cheong, can you help me for the following:
The solution can be found here on Youtube channel "Mind Your Decisions"
Fast answer : 7 9 + 1 0 − 7 2 − 8
The key is to find a triangle or a set of triangles which equal to half of the parallelogram's area.
We will use the following principle:
We will be using the same technique in our problem.
First, let us label the diagram and then identify this:
Then we apply the principle:
Now we can form an equation by making their areas equal:
x + a + 7 2 + b + 8 = a + 7 9 + b + 1 0 ∴ x = 7 9 + 1 0 − 7 2 − 8 = 9