Disk Sections

Geometry Level 3

The inner black circle has $\frac{1}{3}$ the radius of the larger outer circle. Six chords are positioned symmetrically within the larger circle such that they are all tangent to the inner circle.

Which is larger, the $\color{#D61F06}\text{red}$ area or the $\color{#3D99F6}\text{blue}$ area?

Red Blue They're the same size

2 solutions

Brack Harmon
Jun 8, 2018

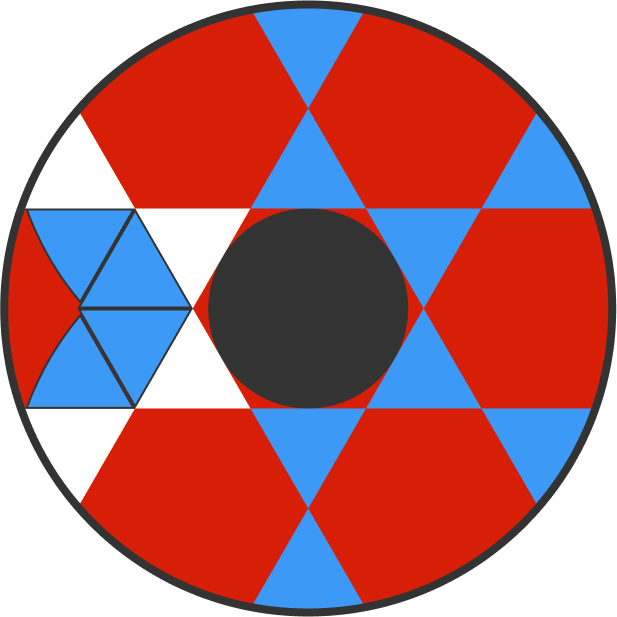
The blue pieces fit inside of the red pieces leaving extra room so the red area is larger.

Hello Brack,

Just a heads up that we changed this problem so that the figure is drawn to scale. This makes your solution out of date, so I wanted to give you a chance to edit it or delete it.

I'm wondering -- did you intend the smaller circle to be $\frac{1}{4}$ the radius of the larger circle (instead of $\frac{1}{3}$ )?

Andrew Hayes Staff - 2 years, 12 months ago

Thank you for making the figure drawn to scale. Also I did intend for the smaller circle to be $\frac{1}{3}$ the radius of the larger circle because I felt $\frac{1}{2}$ was too big.

Brack harmon - 2 years, 12 months ago

Andrew Hayes Staff
Jun 15, 2018

We can flip in the blue regions into the red regions like so:

We would then have to skip every other red area, and we wouldn't even cover them completely! Therefore, the red area is larger.

Disk Sections

2 solutions

0 pending reports