Displacement current in a capacitor
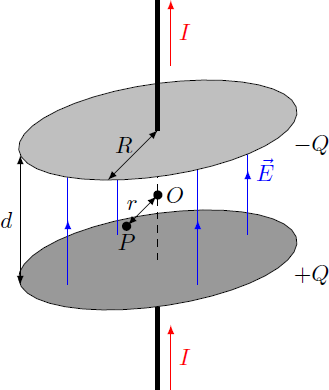
An air capacitor is charged with a constant electric current . What is the magnitude of the induced magnetic field at the point midway between the metal plates at the radial distance from the center (point )? Specify your answer in units of microtesla ( T) with an accuracy of two decimal places.
The capacitor consists of two parallel, circular metal plates with radius at a distance from each other.
Hint: You might use Ampere's law with the current density , the electric field , the permeability and the permittivity .
The answer is 5.00.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
According to Gauss' law, the homogeous electric field inside the capacitor is ∮ E ⋅ d A ⇒ E = E ⋅ π R 2 = ε 0 Q = ε 0 π R 2 Q = ε 0 π R 2 Q 0 + I ⋅ t with the initial charge Q 0 at t = 0 . Inside the capacitor there is no electric current ( j = 0 ), but there is temporal change ∂ t ∂ E of the electric field (displacement current) thereby inducing a magnetic field. Since the capacitor has a rotatianal symmetry, the magnetic field has the form B ( r , t ) = B ( r , t ) ( − sin ϕ cos ϕ ) = B ( r , t ) e ϕ with the unit vector e ϕ ⊥ r . Integrating Ampere's law over a circle with radius r results ∫ ( ∇ × B ) ⋅ d A ⇒ B = ∮ B ⋅ d l = B ⋅ 2 π r = μ 0 ε 0 ∫ ∂ t ∂ E ⋅ d A = μ 0 ε 0 ∂ t ∂ E π r 2 = 2 1 μ 0 ε 0 r ∂ t ∂ E = 2 π R 2 μ 0 I r Using the given numerical values, we get the result B = 2 π ⋅ 4 ⋅ 1 0 − 4 4 π ⋅ 1 0 − 7 ⋅ 1 ⋅ 1 0 − 2 T = 2 1 1 0 − 5 T = 5 μ T