Distance = Speed $\times$ time, right?

Calculus Level 4

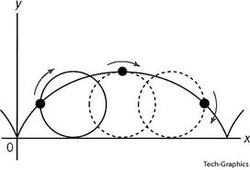A disc is performing pure rolling on a rough and perfectly horizontal surface.

The locus of any point on its circumference is a cycloid.

Find the length of this cycloid corresponding to one revolution of the disc of radius $2 \text{ m}$ .

2 solutions

Vijay Raghavan
Mar 5, 2014

The parametric equations of the cycloid are :

$x = r (t -\sin t)$

$y= r (1- \cos t)$

Now, the arc length of the cyloid is given by :

$\int_0^{2\pi} \sqrt{1+{(\dfrac{dy}{dx}})^2}\, dx$

$= \boxed {8r}$

$= \boxed {16}$ .

Nice one.

Shantanu Nathan - 7 years, 3 months ago

Jaivir Singh
Apr 16, 2014

total distance travell is 8R