Distribution Substation

Note: This problem is meant to be very easy, but there is a lot of text just to provide background

A three-phase distribution substation steps voltage down from $69 \, \text{kV}$ to $12.5 \, \text{kV}$ . These voltages are AC, RMS, line-to-line. The unit $\text{kV}$ denotes one thousand volts.

The step-down transformer passes a total three-phase apparent power of $28 \, \text{MVA} = 28 \times 10^6 \, VA$ , where "VA" stands for "volt-amperes". A single-line diagram is shown for convenience.

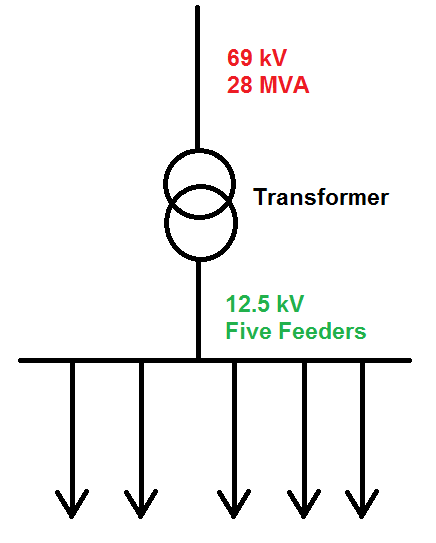
Five identical $12.5 \, \text{kV}$ distribution feeders are fed from a common bus on the low-voltage side of the transformer. Assuming the transformer to be lossless for simplicity, each distribution feeder takes one fifth of the total apparent power flowing through the transformer.

The following expression relates the total three-phase apparent power $S$ on each feeder to the feeder line-to-line voltage $V_{LL}$ and per-phase feeder current $I_L$ .

$S = \sqrt{3} \, V_{LL} \, I_L$

Note that the units of $S$ in the equation are volt-amperes, the units for $V_{LL}$ are volts RMS, and the units for $I_L$ are amps RMS. All quantities in the equation are scalars (real numbers). A current of magnitude $I_L$ flows in each of the three phases.

For each feeder, what is the value of $I_L$ ?