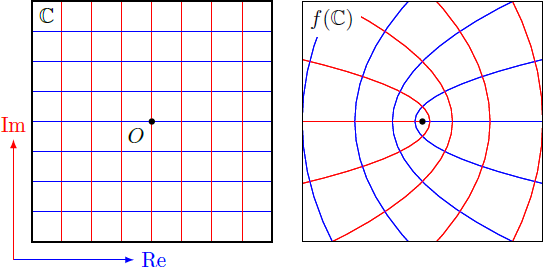
Do you know my function?

$\begin{aligned} f(z) &= \frac{1}{2} z^2 \\ &= \frac{1}{2} (x + i y)^2 \\ &= \frac{1}{2} (x^2 - y^2) + i x y \end{aligned}$ Coordinate lines $x = \text{const}$ and $y = \text{const}$ are parabolas or square roots. For the example $y = 1$ gives the image of the coordinate line $(u, v) = (\frac{1}{2} (x^2 - 1), x)$ , such that $v = \pm \sqrt{2 u + 1}$ . $\begin{aligned} f(z) &= \left(\frac{1}{2} + 2 i \right) z \\ &= \left(\frac{1}{2} + 2 i\right) (x + i y) \\ &= \left(\frac{1}{2}x - 2 y\right) + i \left(2 x + \frac{1}{2} y\right) \end{aligned}$ Coordinate lines remain straight lines. The coordinate net is only rotated and stretched by a constant factor. $\begin{aligned} f(z) &= \frac{1}{z} \\ &= \frac{1}{x + i y} \\ &= \frac{1}{x + i y} \cdot \frac{x - i y}{x - i y} \\ &= \frac{x}{x^2 + y^2} - \frac{i y}{x^2 + y^2} \end{aligned}$ The complex plane is virtually inverted by this mapping. Points at infinity are mapped to the origin so that all coordinate lines intersect here. In addition, all coordinate lines are mapped to circles (can you proof this?). $\begin{aligned} f(z) &= \cos(z) \\ &= \frac{1}{2} (\text{e}^{i (x + i y)} + \text{e}^{-i (x + i y)}) \\ &= \frac{1}{2} (\text{e}^{-y + i x} + \text{e}^{y - i x}) \\ &= \frac{1}{2} (\text{e}^{-y}(\cos(x) + i \sin(x)) + \text{e}^{y} (\cos(x) - i \sin(x))) \\ &= \cos(x) \cosh(y) - i \sin(x) \sinh(y) \end{aligned}$ The coordinate lines $y = \text {const}$ are ellipses with semi-axes $a = \cosh (y)$ and $b = \sinh (y)$ .