Do you remember Circles?

If O be the centre of the circle then triangle AOC is equilateral and hence / AOC=60° which makes / ABC=150. This should be a Level 1 problem with a lesser weightage.

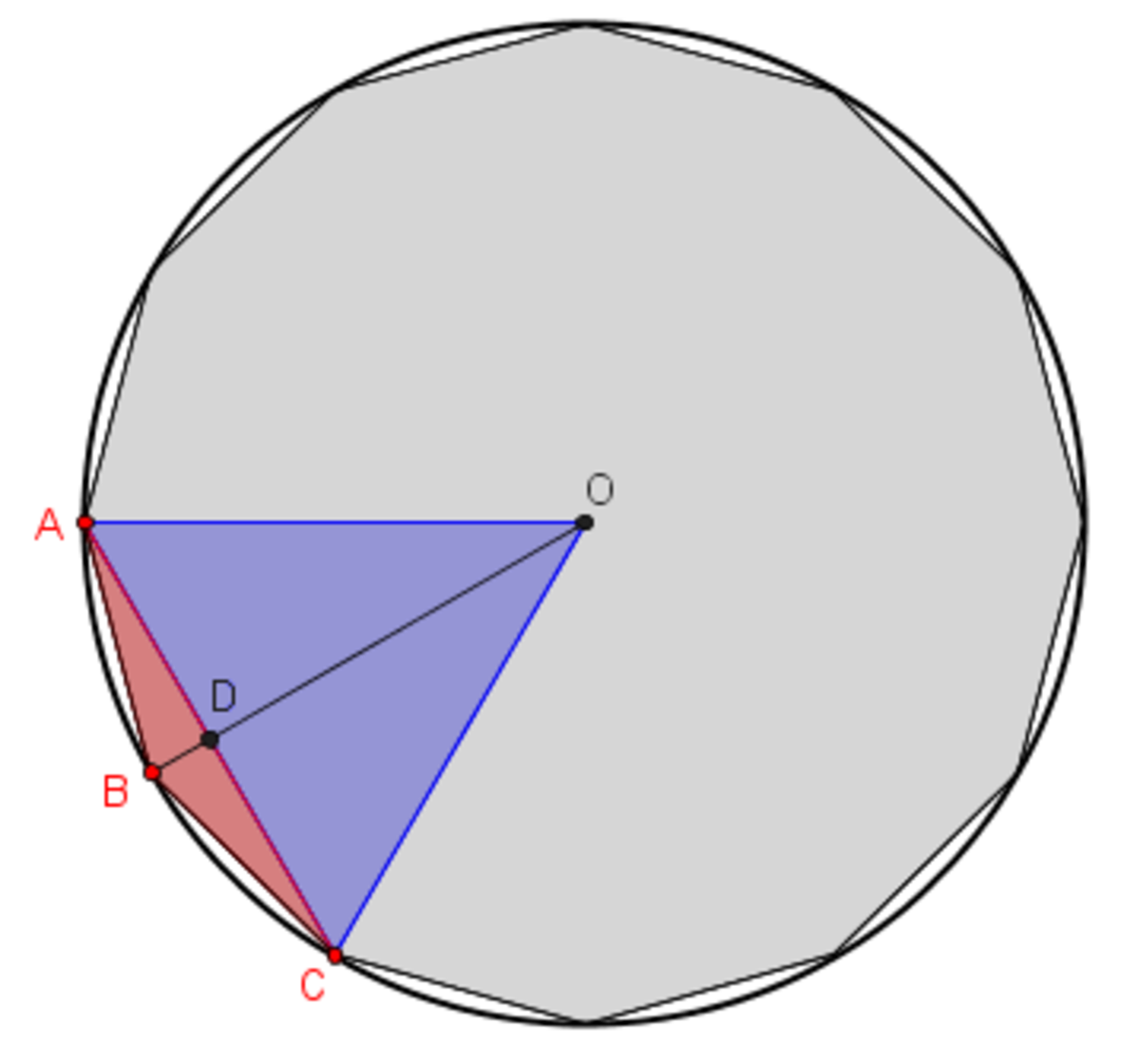
$\color{#3D99F6}{\triangle OAC}$ is equilateral and $OD$ is a perpendicular bisector to chord $AC$ . $\space \overline{OD}$ is extended to point $B$ so points $O,D,B$ are collinear. $\space \triangle ABD \cong \triangle CBD$ by SAS congruence. This proves $AB \cong CB$ citing corresponding parts of congruent triangles. $\space \color{#D61F06}{\triangle ABC}$ is therefore isosceles. We can now conclude that we just inscribed $\frac{1}{6}$ of a regular polygon inside the circle (i.e., $\frac{60^{\circ}}{360^{\circ}}$ ). $\space \angle ABC$ is therefore the interior angle of a regular dodecagon since we can repeat this construction 6 times in total.

$\therefore 150^{\circ}$